Students of ICSE Class 10 should refer to Force ICSE Class 10 Physics Questions below which have come in past board exams. You should always go through questions that have come in previous years. This will help you to understand the pattern of questions in ICSE Class 10 Physics and prepare accordingly. This will help you to get better marks in ICSE Class 10 Board Exams
ICSE Class 10 Physics Force Important Questions
Students should learn the important questions and answers given below for Chapter Force in Physics for ICSE Class 10. These board questions are expected to come in the upcoming exams. Students of ICSE Class 10th should go through the Important questions and answers ICSE Class 10 Physics which will help them to get more marks in exams.
Force ICSE Class 10 Physics Questions
SCOPE OF SYLLABUS
Elementary introduction of translational and rotational motions: moment (turning effect) of a force, also called torque and its C.G.S. and S.I. units, common examples – door, steering wheel, bicycle pedal, etc., clockwise and anticlockwise moments, conditions for a body to be in equilibrium (translational and rotational), principle of moments and its verification using a metre rule suspended by two spring balances with slotted weights hanging from it, simple numerical problems, centre of gravity (qualitative only) with examples of some regular bodies and irregular lamina.
MOTION AND MOMENT OF A FORCE
1. State the condition when a force produces :
(a) translational motion
(b) rotational motion
Ans.
(a) When the body is free to move
(b) When the body is pivoted at a point.
2. Define moment of force and state its S.I. units.
Ans. The turning effect of force acting on a body about an axis is called the moment of force.
S.I. unit : Nm (newton metre)
3. Is moment of a force a scalar or a vector?
Ans. Moment of force is a vector quantity.
4. State two factors on which moment of force about a point depends.
Ans. (i) The magnitude of the force applied
(ii) The perpendicular distance of line of action of the force from the axis of rotation.
5. When does a body rotate? State one way to change the direction of rotation of body. Give a suitable example to explain your answer.
Ans. A body pivoted at point O, when the force is applied on the body at a suitable point, it rotates the body about the axis passing through the pivoted point.
This is called rotational motion.
Example: When a force is applied normally on the handle of a door, the door begins to rotate about an axis passing through the hinges on which the door rests.
To change the direction of rotation: For turning a steering wheel anticlockwise, the force is applied tangentially at A and to change the direction of motion i.e. to turn clockwise, the force F is applied at B. In other words the point of application is changed.
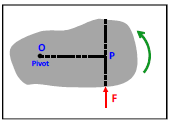
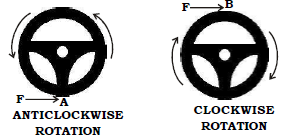
Sense of rotation changed by the change of point of application of force.
5. Write the expression for calculating the moment of force about a given axis.
Ans. Moment of force = F × perpendicular distance of force from the axis of rotation.
6. State one way to reduce the moment of given force about a given axis of rotation.
Ans. Increase the distance between line of action and axis of rotation.
E.g. : In a see-saw, a man sitting on one side of side of it is balanced by a child when the child sits at a greater distance from the axis of rotation on the other side.
7. State one way to obtain a greater moment of force about a given axis of rotation.
Ans. The force is applied at a point on the body for which the perpendicular distance of line of action of the force from axis of rotation is maximum so that the given force may provide the maximum torque to turn the body.
8. What do you understand by the clockwise and anticlockwise moment of force?
When is it taken positive?
Ans. Clockwise moment – When the moment of force is in the clockwise direction.
Anticlockwise moment – When the moment of force acts in anticlockwise direction.
Anticlockwise moment of force is positive.
9. Why is it easier to open a door by applying the force at the free end of it?
Ans. Moment of force is product of force and perpendicular distance, hence it is easier to open the door by applying force at the handle.
10. The stone of hand flour grinder is provided with a handle near its rim. Give reason.
Ans. In order to increase of moment of force, handle is provided near its arm.
11. It is easier to turn the steering wheel of a large diameter than that of a small diameter. Give reason.
Ans. The hand of large diameter has a large perpendicular distance, the moment of force depends upon perpendicular distance.
12. A spanner (or wrench) has a long handle. Why?
Ans. Spanner has a long handle to produce larger turning moment. The force is appliedat the end of handles.
13. A, B and C are three forces each of magnitude 4 N acting in the plane of paper as shown in the figure. The point O lies in the same plane
(i) Which force has the least moment about O? Give reason.
(ii) Which force has the greatest moment about O? Give reason.
(iii) Name the force producing (a) clockwise (b) anticlockwise moments.
(iv) What is the resultant torque about the point O?
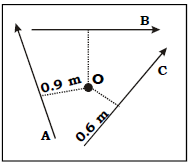
Ans. (i) C, because force C is nearest to O.
(ii) A, because force, A is farthest from O.
(iii) (a) A and B are producing clockwise moments.
(b) C force is producing anticlockwise moment.
(iv) The clockwise moment of force about O = Force × OA = 4N × 0.9m = 3.6 Nm
The clockwise moment of force about O = Force × OB = 4N × 0.8m = 3.2 Nm
Total clockwise moment of the two forces about O = (3.6 + 3.2) Nm = 6.8 Nm
The anticlockwise moment of force about O = Force × OB = 4N × 0.6m = 2.4 Nm
The resultant torque about O = anticlockwise moments + clockwise moments
= +2.4 Nm + (-6.8) Nm
= 2.4 Nm – 6.8 Nm = -4.4Nm
= 4.4 Nm (clockwise)
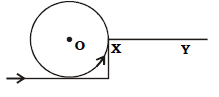
14. The adjacent diagram shows a heavy roller, with its axle at O, which is to be raised on a pavement XY by applying a minimum possible force. Show by the direction in which the force should be applied.
Ans.
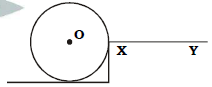
15. A body is acted upon by two forces each of magnitude F, but in opposite directions. State the effect of the forces if :
(a) both forces act at the same point of the body.
(b) the two forces act at two different point of the body at a separation r.
Ans. (a) Resultant force = 0, Moment of force = 0 . No motion
(b) The forces tend to rotate the body about the mid point between the two forces, Resultant force = 0, Moment of force = Force × r.
16. Draw a neat labelled diagram to show the direction of two forces acting on a body to produce rotation in it. Also mark the point about which rotation takes place, by the letter O.
Ans.
COUPLE AND MOMENT OF COUPLE
1. What do you understand by the term couple? State its effect. Give two examples of couple action in our daily life.
Ans. Two equal and opposite parallel forces not acting along the same line form a couple.
A greater force acts on it.
(i) opening of a door
(ii) turning of a water tap.
2. Define moment of couple. Write its S.I unit.
Ans. Moment of a couple is equal to the product of either force and the perpendicular distance between the line of action of the both the forces.
S.I. unit : Nm
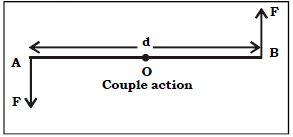
3. Prove that : Moment of couple = Force × Couple arm.
Ans.Moment of force F at the end A = F × OA (anticlockwise)
Moment of force F at the end B = F × OB (anticlockwise)
Total moment of couple
= F × OB + F × OB
= F (OA + OB)
= F × AB
= Force × Couple arm
EQUILIBRIUM
1. What do you mean by equilibrium of a body?
Ans. When a number of forces acting on a body produce no change in its state of rest or of motion, the body is said to in equilibrium.
2. State the condition when a body is in (i) static equilibrium, (ii) dynamic equilibrium. Give one example each of static and dynamic equilibrium.
Ans. (i) The resultant of all the forces acting on a body should be equal to zero.
(ii) The resultant moment of all the forces acting on the body about the point of rotation should be zero.
(i) Example of static equilibrium – The book lying on a table. The weight of book exerted on the table vertically
downwards is balanced by the equal and opposite force of reaction exerted by the table on the book vertically
upward. Thus, the book is in static equilibrium.
(ii) Example of Dynamic equilibrium – A planet moving around the sun is in a state of dynamic equilibrium because the force of attraction on planet by the sun provides the centripetal force. Thus, the planet is in dynamic equilibrium
3. State two conditions for a body acted upon by several forces to be in equilibrium
Ans. (i) The resultant of all the forces acting on the body should be equal to zero.
(ii) The resultant moment of all the forces acting on the body about the point of rotation should be zero.
PRINCIPLE OF MOMENTS
1. State the principle of moments. Give one device as application of it.
Ans. According to principle of moments, in equilibrium the sum of anticlockwise moments is equal to the sum of the clockwise moment.
A physical balance works on the principle of moments.
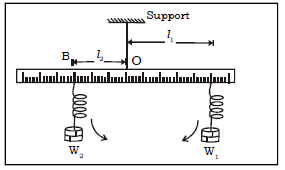
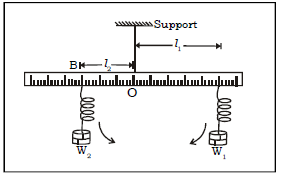
2. Describe a simple experiment to verify the principle of moments, if you are supplied with a metre rule, a fulcrum and two springs with slotted weights.
Ans. Suspend a metre scale horizontally from a fixed support by means of a strong thread. Now suspend the two spring balances with some slotted weights W1 and W2 on either side. One at A and other at B by adjusting their distances in such a way that the scale becomes horizontal.
Let the distance OA = l1 and distance OB = l2
The weight W1 tend to turn the scale clockwise, while the weight W2 tend to turn the scale anticlockwise.
Clockwise moment = W1 × l1
Anticlockwise moment = W2 × l2
According to principle of equilibrium,
Sum of clockwise moment = Sum of anticlockwise moments
W1 × l1 = W2 × l2
10. A uniform flat circular rim is balanced on a sharp vertical nail by supporting it at point A, as shown in figure Mark the position of centre of gravity of the rim in the diagram by the letter G.
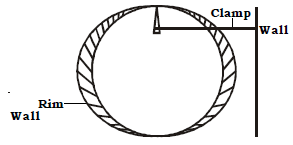
Ans.
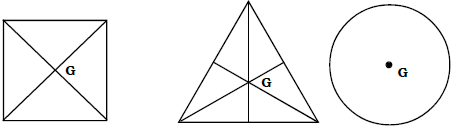
11. Figure shows three pieces of card board of uniform thickness cut into different shapes. On each diagram draw two lines to indicate the position of centre of gravity G.

Ans.
MULTIPLE CHOICE QUESTION
MULTIPLE CHOICE QUESTIONS
1. The moment of a force about a given axis depends:
(a) Only on the magnitude of force
(b) Only on the perpendicular distance of force from the axis
(c) Neither on the force nor on the perpendicular distance of force from the axis
(d) Both on the force and its perpendicular distance from the axis.
Ans. (b) Both on the force and its perpendicular distance from the axis.
2. The unit of moment of force is:
(a) N
(b) Nm–1
(c) kg m2 s-1
(d) N m
Ans. (d) N m
3. In order to rotate a bar pivoted at its midpoint we need to apply:
(a) A large force at any point on the bar
(b) A small force exactly at the mid point where it is pivoted.
(c) Two unequal forces at the two ends, but in the same direction.
(d) Two equal forces at the two ends, but in opposite directions.
Ans. (d) Two equal forces at the two ends, but in opposite direction.
4. A body is acted upon by two unequal forces in opposite directions, but not in one line. The effect is that:
(a) The body will have only the rotational motion
(b) The body will have only the translational motion.
(c) The body will have neither the rotational notion nor the translational motion.
(d) The body will have rotational as well as translation motion.
Ans. (d) The body will have rotational as well as translation motion.
5. The appliance which works on the principle of moments, is:
(a) Spring balance (b) Beam balance
(c) pendulum clock (d) Balance wheel.
Ans. (b) Beam balance
NUMERICALS BASED ON MOMENT OF FORCE & PRINCIPLE OF MOMENTS
1. The moment of a force of 10 N about a fixed point O is 5 Nm. Calculate the distance of the point O from the line of action of the force.
Ans. Moment of force = 5 N m, Force = 10 N, Distance = ?
Moment of force = Force × Distance
5 = 10 × Distance
Distance = 5/10 = 0.5m
2. A nut is opened by a wrench of length 10cm. If the least force required is 5.0 N, find the moment of force needed to turn the nut.
Ans. Distance = 10 cm = 0.1m, Force = 5.0 N, Moment of force = ?
Moment of force = Force × Distance
Moment of force = 5 × 0.1 = 0.5 N m
3. A wheel of diameter 2 m is shown in figure with axle at O. A force of 2N is applied at B in the direction shown in figure. Calculate the moment of force about (i) centre O (ii) point A.
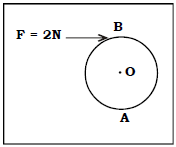
Ans. Diameter of wheel = 2m
Radius of wheel =2/2=1
Force applied at B = 2 N
(i) Moment of force at O (clockwise) = 2 × 1 = 2N m
(ii)Moment of force at A (clockwise) = 2 × 2 = 4N m
4. The diagram in figure shows two forces F1 = 5 N and F2 = 3 N acting at points A and B of a rod pivoted at a point O, such that OA = 2m and OB = 4m.
Calculate:
(i) Moment of force F1 about O.
(ii) Moment of force F2 about O.
(iii) Total moment of the two forces about O.
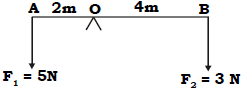
Ans. (i) Moment of force F1 about O = Force × AO
= 5 × 2
= 10 Nm (anticlockwise)
(ii) Moment of force F2 about O = Force × BO
= 3 × 4
= 12 Nm (anticlockwise)
(iii) Total moment = Clockwise Moments + Anticlockwise Moments
= –12 + 10
= –2Nm = 2Nm (clockwise)
5. Two forces each of magnitude 10 N act vertically upwards and downwards respectively on the two ends of a uniform rod of length 4 m which is pivoted at its mid point as shown in figure. Determinethe magnitude of resultant moment of forces about the pivot O.
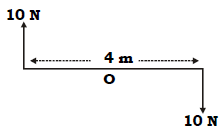
Ans. F = 10 N on each side
Distance of each force from O = 2m
Both are clockwise moments,
Resultant moment = 10 × 2 + 10 × 2 = 20 + 20 = 40 Nm (Clockwise)
6. Figure shows two forces each of magnitude 10 N acting at the points A and B at a separation of 50cm, in opposite directions. Calculate the resultant moment of the two forces about the point
(i) A
(ii) B
(iii) O, situated exactly at the middle of the two forces.
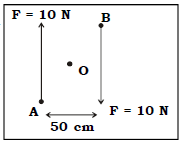
Ans. Moments of force = Force × Distance
F = 10 N at A and B
Distance between them = 50 cm = 0.5m
Moment of force at A = 10 × 0.5 = 5 Nm (clockwise)
Moment of force at B = 10 × 0.5 = 5 Nm (clockwise)
Moment of force at O = 10 × 0.25 + 10 × 0.25 = 5 Nm(clockwise)
7. A steering wheel of diameter 0.5 m is rotated anticlockwise by applying two forces each of magnitude 5N. Draw a diagram to show the application of forces and calculate the moment of couple applied.
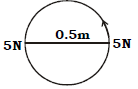
Ans. Diameter of steering wheel= 0.5m
Force = 5 N
Moment of couple = Either Force × Perpendicular distance
Moment of couple = 5 × 0.5
= 2.5 Nm (Anticlockwise)
8. A uniform metre rule is pivoted at its mid-point. A weight of 50gf is suspended at one end of it. Where should a weight of 100 gf be suspended to keep the rule horizontal?
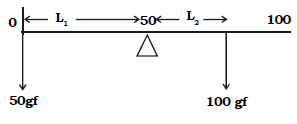
Ans. L1 = 50 cm
W1 = 50gf
W2 = 100gf
L2 = ?
By Principle of Moments,
W1 × L1 = W2 ×L2
50 × 50 = 100 × L2
50 x 50 / 100 = L2 = 25 cm
9. A uniform metre rule balances horizontally on a knife edge placed at the 58 cm mark when a weight of 20 gf is suspended from one end.
(i) Draw a diagram of the arrangement.
(ii) What is the weight of the rule?
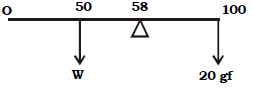
Ans. W1 = 20gf L1 = 42cm
W2 = Wgf L2 = 8cm
By Principle of Moments,
W1 × L1 = W2 × L2
20 × 42 = W × 8
W =20 x 42 / 8
W = 105 gfcm
10. The diagram shows a uniform bar supported at the middle point O.A weight of 40 gf is placed at a distance 40 cm to the left of the point O. How can you balance the bar with a weight of 80gf ?
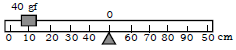
Ans. W1 = 40gf , W2 = 80gf
d1 = 40cm , d2 = ?
W1 × d1 = W2 × d2
40 × 40 = 80 × d2
d2 =40 × 40 / 80 = 20cm
By placing the weight 80 gf at a distance 20 cm to the right of the point O.
11. Figure shows a uniform metre rule placed on a fulcrum at its mid-point O and having a weight 40 gf at the 10 cm mark and a weight of 20 gf at the 90 cm mark.(i) Is the metre rule in equilibrium ? if not, how will the rule turn? (ii)How can the rule be brought in equilibrium by using an additional weight of 40 gf ?
Ans. W1= 40gf , W2 = 20gf
L1 = 40cm , L2 = 40cm
Anticlockwise Moment = W1 × L1
= 40 × 40
= 1600gf cm
Clockwise Moment = W2 × L2
= 40 × 20
= 800gf cm
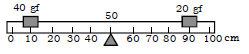
(i) No. It turns in anticlockwise direction
(ii)Difference in moment of force= Anticlockwise moment + Clockwise moment
= 1600 + (- 800)
= 800gf cm
Moment of Force = F × r
800 = 40 × r
r = 800 / 40 = 20
Rule is in equilibrium when additional weight is placed at 70 cm mark from the fulcrum.
12 . When a boy weighing 20kgf sits at one end of a 4 m long see-saw, it gets depressed at this end. How can it be brought to the horizontal position by a man weighing 40kgf.
Ans. W1 = 20kgf , W2 = 40kgf
Length of see-saw = 4m (Pivated at its midpoint)
d1 = 2m
W1 × d1= W2 × d2
20 × 2 = 40 × d2
d2 =20 x 2 / 40= 1 m
By sitting at a distance 1m from the centre on the side opposite to the boy
13. A physical balance has its arms of length 60cm and 40cm. What weight kept on pan of longer arm will balance an object of weight 100 gf kept on other pan?
Ans. l1 = 60cm, l2 = 40cm, w2= 100 kgf on shorter length, w1 = ?
By Principle of moments,
w1 × l1= w2 × l2
w1 × 60 = 100 × 40
w1 = 100 x 40 / 60 = = 66.67gf
14. The diagram shows a uniform metre rule weighing 100gf, pivoted at its centre O. Two weights 150 gf and 250 gf hang from the metre rule as shown. Calculate:
(i) The total anticlockwise moment about O
(ii) The total clockwise moment about O
(iii) The difference of anticlockwise and clockwise moment
(iv) The distance from O where a 100 gf weight should be placed to balance the metre rule.
Ans. (i) Anticlockwise moment = 150 × 40 = 6000gf cm
(ii) Clockwise moment = 250 × 20 = 5000gf cm
(iii) Difference of anticlockwise moments and Clockwise moment = 6000 – 5000
= 1000gf cm (anticlockwise)
(iv) Moment of Force = F × r
1000 = 100 × r
r = 10cm to the right of O
15. A uniform metre rule of weight 10 gf is pivoted at its 0 mark
(i) What moment of force depresses the rule?
(ii) How can it be made horizontal by applying a least force?
Ans. Weight of metre scale = 10gf
(i) Moment of force = 10 × 50 = 500gf cm
(ii) It can be made horizontal by moment of force = 500 gf cm
500 = w ×100
w = 500 / 100 = 5gf
By applying 5gf upwards at the 100cm mark
16. A uniform metre scale can be balanced at the 70.0cm mark when a mass 0.05kg is hung from 94.0cm mark.
(a) Draw a diagram of the arrangement.
(b) Find the mass of metre scale.
Ans. (a)
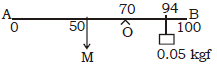
(b) Uniform metre scale AB rests on the knife edge at O. Let M be the weight of scale.
A uniform scale has same distribution of mass throughout its length. So the weight M will act at its middle point i.e. at 50cm mark .
By the principle of moments,
Anticlock wise moment = clockwise moment
M × (70 – 50) = 0.05 (94 – 70)
20M = 0.05 × 24
M =0.05 × 24 / 20 = 0.06kgf
–Mass of the scale is 0.06kg
17. A uniform metre rule of mass 100g is balance on a fulcrum at mark 40 cm by suspending an unknown mass m at the mark 20cm.
(i) Find the value of m.
(ii) To which side the rule will tilt if the mass m is moved to the mark 10cm?
(iii) What is the resultant moment now?
(iv) How can it be balanced by another mass 50g?
Ans. (i) The weight 100 g of rule produces clockwise moment about knife edge O.
Weight m produces anticlockwise moment.
From the principle of moments,
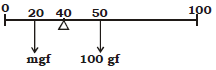
Anticlockwise moment = clockwise moment
(40 – 20) m = 100 (50 – 40)
20m = 100 × 10
m =100 × 10 / 20 = 50gf
Mass is 50 g.
(ii) If mass m is moved to 10cm mark i.e. to the left side, the rule will tilt to left side i.e. to the side of m.
Anticlockwise moment = 50 × 30 = 1500 gfcm
Clockwise moment = 100 × 10 = 1000gfcm
Resultant moment = + 1500 – 1000 = 500 gfcm (anticlockwise)
(iv) Let mass of 50 g be suspended at a distance of x cms from O to the right side to balance the weight of 50gf at 10cm mark.
– Clockwise moment = Anticlockwise moment
100 × 10 + 50x = 50 × 30
50x = 1500 – 1000 = 500
x =500/50 = 10cm to the right from 40 cm mark i.e.at 50 cm mark.
18. In the figure, a uniform bar of length l m is supported at its ends and loaded by a weight W kgf at its middle. In equilibrium, find the reactions R1 and R2 at the ends.
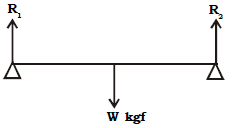
Ans. As D is the mid point of AB (l)
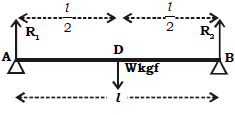
∴ AD = DB = l/2
Weight W of the bar acts downward towards the centre of earth and normal rections R1 and R2 acting in upward direction balance the weight W.
∴ In equilibrium R1 + R2 = W
Total upward reaction = (R1 × AD) + (R2 × DB)
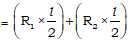
R1 ×l/2= R2 ×l/2= R (suppose)
Total upward reaction = downward weight of bar
∴ R + R = W
2R = W
R = W/2
R1 = W/2 kgf and R2 = W / 2 kgf
CENTRE OF GRAVITY
1. Define the term ‘centre of gravity of a body’.
Ans. Centre of gravity of a body is the point through which the entire weight of body acts howsoever it is placed.
2. Can the centre of gravity be situated outside the material of the body? Give an example.
s. Yes. For example, C.G of a ring or a hollow sphere.
3. On what factor does the position of centre of gravity of a body depend? Explain your answer with an example.
Ans. (i) The position of centre of gravity of a body of given mass depends on its shape
i.e. on the distribution of mass in it
(ii) It is not necessary that centre of gravity always be within the material of the body. For Ex : A ring.
4. What is the position of centre of gravity of a : (a) rectangular lamina (b) cylinder?
Ans. (a) At the point of intersection of its diagonals.
(b) Mid-point of the axis of cylinder
5. At which point is the centre of gravity situated in (a) a triangular lamina and (b) a circular lamina?
Ans. (a) At the point of intersection of its medians
(b) Centre of circular lamina.
6. Where is the centre of gravity of a uniform ring situated?
Ans. At the centre of ring.
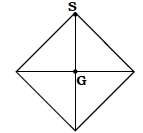
7. A square card board is suspended by passing a pin through a narrow hold at its one corner. Draw a diagram to show its rest position. In the diagram, mark the point of suspension by the letter S and centre of gravity by the letter G.
Ans.
8. Explain how you will determine experimentally the position of centre of gravity for a triangular lamina (or a triangular piece of card board).
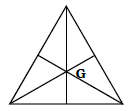
Ans. Take a triangular lamina. Draw its medians where the medians intersect that will be centre of gravity of triangular lamina.
9. State whether the following statement is true or false.
(i) ‘The position of centre of gravity of a body remains unchanged even when the body is deformed.’
(ii) ‘Centre of gravity of a freely suspended body always lies vertically below the point of suspension.’
Ans. (i) False
(ii) True
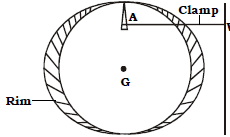
10. A uniform flat circular rim is balanced on a sharp vertical nail by supporting it at point A, as shown in figure Mark the position of centre of gravity of the rim in the diagram by the letter G.

Ans.

11. Figure shows three pieces of card board of uniform thickness cut into different shapes. On each diagram draw two lines to indicate the position of centre of gravity G.

Ans.

MULTIPLE CHOICE QUESTION
1 . The incorrect statement is:
(a) A body can be balanced at its centre of gravity.
(b) The total weight of a body acts at its centre of gravity.
(c) The algebraic sum of moments of the weights of all particles constituting a body is zero about its centre of gravity.
(d) The centre of gravity of a body depends on how it is placed.
Answer
(d) The centre of gravity of a body depends on how it is placed.
2. The centre of gravity of a uniform ball is
(a) At its geometrical centre
(b) At its bottom
(c) At its topmost point
(d) At any point on its surface
Answer
(a) At its geometrical centre
UNIFORM CIRCULAR MOTION
1. Explain the meaning of uniform circular motion. Give one example of such motion.
Ans. When a particle moves with a constant speed in a circular path, its motion is said to be uniform circular motion. E.g : Revolution of moon around the Earth.
2. Draw a neat labelled diagram for a particle moving in a circular path with a constant speed. In your diagram show the direction of velocity at any instant.
Ans.
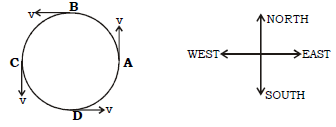
3. Is it possible to have an accelerated motion with a constant speed? Name such type of motion.
Ans. Yes, uniform circular motion.
4. Give an example is motion in which speed remains uniform, but the velocity changes.
Ans. When the moon is revolving around the Earth, the speed remains uniform, but since the direction changes every instant, hence the velocity changes at every instant.
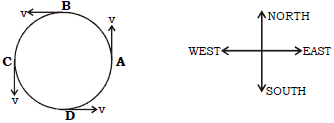
5. A uniform circular motion is an accelerated motion. Explain it. State whether the acceleration is uniform or variable? Name the force responsible to cause this acceleration. What is the direction of force at any instant? Draw diagram in support of your answer.
Ans. When a particle moves with Uniform circular motion (circular path), the speed of particle is uniform but direction of motion changes at each point of path i.e., velocity of particle is variable and hence the motion is accelerated. Acceleration is variable.
The force responsible to cause this motion is centripetal force.
The direction of force at any instant is directed towards the centre of the circle.

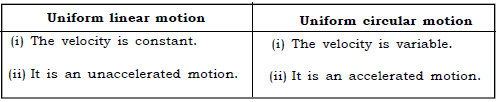
6. Differentiate between a uniform linear motion and a uniform circular motion.
Ans.
7. Name the force required for circular motion. State direction.
Ans. Centripetal force towards the centre.
8. What is centripetal force?
Ans. The motion in a circular path is under the action of a force which is called as acting towards the centre is centripetal force.
9. A piece of stone tied at the end of a thread is whirled in a horizontal circle.
Name the force which provides the centripetal force.
Ans. Force of tension in the thread.
10. Explain the motion of a planet around the sun in a circular path.
Ans. A planet moves around the sun in a circular path for which the force of attraction on the planet by the sun provides the necessary centripetal force.
11. (a) With reference to the direction of action, how does a centripetal force differ from a centrifugal force?
Ans. The centrifugal force is in direction opposite to the centripetal force, Centripetal force is always directed towards the centre of circle whereas the centrifugal force acts on a body away from the centre of circular path.
(b) Is centrifugal force the force of reaction of centripetal force?
Ans. No.
(c) Compare the magnitudes of cemtripetal and centrfugal force .
Ans. 1 : 1
12. Is centrifugal force a real force?
Ans. No.
13. A small pebble is placed near the periphery of a circular disc which is rotating about an axis passing through it centre.
(a) What will be your observation when you are standing outside the disc?
Explain it.
(b) What will be your observation when you are standing at the centre of the disc. Explain it.
Ans. (a) While you stand outside the disc, a small pebble which is placed near the circular disc, which is rotating on its axis, the pebble all seems to moving in circular path.
(b) When you are standing at the centre of disc, it is seen that the pebble is moving away from you and falls down.
14. State whether the following statements are true or false.
(a) The earth moves around the sun with a uniform velocity.
(b) The motion of the moon around the earth in circular path is an accelerated motion.
(c) A uniform linear motion, is unaccelerated, while a uniform circular motion is an accelerated motion.
(d) In a uniform circular motion, the speed continuously changes because the direction of the motion changes.
Ans. (a) False (b) True (c) True (d) False
FORMULAE AND RELATIONS
Formulae :-
F = m × a F = m × g
v = u + at s = ut + 1/2 at2 v2 = u2 + 2as
Moment of force = Force × perpendicular Distance.
Moment Couple = Either Force × Perpendicular Distance between two forces
Principle of moments :
Sum of Anticlockwise Moments = Sum of Clockwise Moments
Relations :-
1N = 105 dyne
1kgf = 9.8 N (10 N)
1 Nm = 107 dynecm
Units:-
F = kgms-2 = N
Momentum of Force = N × m
Momentum of couple = N × m
Note :- For using principle of moments , the weight should be in kgf or N or gf or dyne.Remember to convert masses into weight while solving numericals.
Assignment
Q.1. Solve the following :
1. Why do we use a long handle with a screw jack ?
2. Why is it easier to open the cap of a pen with two fingers than with one finger?
3. Draw a diagram and indicate the position of the centre of gravity of
a) rectangular lamina
b) lamina of the shape of a parallelogram
c) triangular lamina
d) a thin disc
e) a cylinder.
4. What do you mean by the terms :
(i) translational motion (ii) rotational motion?
5. Can the centre of gravity of a body be outside it ? If yes, give one example.
6. Name the two units of moment of a force. State the relation between them.
7. Why it is difficult to open the door by pushing it or pulling it at the hinge ? Explain.
8. To pull open a heavy gate using minimum force where and in what direction the force should be applied? Why ?
9. What is meant by equilibrium ? State the necessary conditions for a body to be in equilibrium.
10. Explain briefly the role of the position of centre of gravity in (a) loading a ship (b) sitting/standing on the upper deck of a bus.
11. What is (i) centripetal force (ii) centrifugal force?
Q.2. Solve the following :
1. A uniform metre scale of mass 80 g is to be balanced at the 70 cm mark. Find, from where must a mass of 60 g be suspended to do so.
2. A uniform metre scale is balanced at the 20 cm mark when masses of 100 g and 50 g are suspended from the 10 cm and 30 cm mark respectively. Calculate the mass of the metre scale.
3. The moment of a force of 12 N about a fixed point 0 is 6 Nm. Calculate the distance of the point 0 from the line of action of the force.
4. A uniform metre scale can be balanced at the 70.0 cm mark when a mass of 0.05 kg is hung from 94.0 cm mark
(i) Draw a diagram of the arrangement.
(ii) Find the weight of the metre scale.
5. A uniform metre scale of mass 60 g, carries masses of 20 g, 30 g and 80 g from points 10 cm, 20 cm and 90 cm mark. Where must the knife edge be placed to balance the scale ?
6. On a see-saw, two children of masses 30 kg and 60 kg are sitting on one side of it at distances 2 m and 2.5 m respectively from the centre of the see-saw. Find the distance at which a man of mass 75 kg should sit on the other side to balance the see-saw.
7. A uniform metre scale rests horizontally on a knife edge at the 60 cm mark when a mass of 10 g is suspended from one end. From which end should this mass be suspended ? What is the mass of the metre scale ?
8. A man can open a nut by applying a force of 150 N by using a lever handle of length 0.4 m. What should be the length of the handle if he is able to open it by applying a force of 60 N ?
9. A 50 cm uniform ruler is freely pivoted at 15 cm mark which balances horizontally when an object of weight 40 dyne is hung from the 2 cm mark. Draw a diagram of the arrangement and calculate the weight of the ruler. Also calculate the force with which pivot is pressed.
10. A half metre ruler is suspended by a thread from the mid-point of the ruler as shown in the figure. It balances horizontally when a 50 g and an unknown weight Ware suspended respectively from 10 cm and the 45cm mark. Calculate the magnitude of weight W.
MCQ Questions for Class 10 ICSE Physics Force PDF
Check Our MCQ Questions for Class 10 ICSE Physics Force pdf with Answers free download. ICSE Class 10 Physics Force MCQ Questions are prepared according to the latest exam pattern of Icse boards. We have Provided you Force Class 10 ICSE MCQ Questions Pdf with Answers to make your preparation to score good marks in the Class 10 exam.
Question 1
The moment of a force about a given axis depends:
(a) Only on the magnitude of force
(b) Only on the perpendicular distance of force from the axis
(c) Neither on the force nor on the perpendicular distance of force from the axis
(d) On both the force and its perpendicular distance from the axis.
Answer
D
Question-2 A nut is opened by a wrench of length 10cm. If the least force required is 5.0N, find the moment of force needed to turn the nut.
(a) 0.5 Nm
(b) 50 Nm
(c) 2 Nm
(d) None of above
Answer
A
Question -3 Two forces each of magnitude 10 N act vertically upwards and downwards respectively at the two ends A and B of a uniform rod of length 4 m which is pivoted at its mid-point O as shown. Determine the magnitude of resultant moment of forces about the pivot O.
Two forces each of magnitude 10 N act vertically upwards and downwards respectively at the two ends A and B
(a) 20 Nm (clockwise), 20 Nm (anticlockwise)
(b) 20 Nm (anticlockwise), 20 Nm (clockwise)
(c) 40 Nm (clockwise)
Answer
C
Question 4. The point of action of force on a rigid body is :
(a) Fixed point on rigid body
(b) Fixed point but can be transferred any where along the line of action of force.
(c) Fixed point but can be transferred anywhere along the direction of force.
(d) Fixed point, but can be transferred anywhere opposite to the direction of force.
Answer
B
Question 5. The unit of moment of force in SI system is :
(a) Nm
(b) dyne cm
(c) dyne m
(d) N cm
Answer
A
Question 6 The moment of couple is mathematically the :
(a) product of one force and the perpendicular distance between two forces
(b) product of both forces and the perpendicular distance between them
(c) product of one force and tKe perpendicular distance between the point of application of force and turning point.
(d) None of the above.
Answer
A
Question 7. The condition for equilibrium is:
(a) the resultant of all the forces acting on the body be zero only.
(b) the resultant of moments of all the forces acting on the body about the turning point should be zero.
(c) both (a) and (b)
(d) none of the above
Answer
B
Question -8. The turning effect produced in a rigid body around a fixed point by the application of force is called;
(a) turning force
(b) movement of force
(c) moment of couple
(d) none of these
Answer
B
Question-9
In figure below, a uniform bar of length l m is supported at its ends and loaded by a weight W kgf at its middle. In equilibrium, find the reactions R1 and R2 at the ends.
a uniform bar of length l m is supported at its ends and loaded by a weight W kgf at its middle. In equilibrium, find the reactions R1 and R2 at the ends.
(a) w/2 kgf
(b) 2w kgf
(c) R1 + R2 kgf
(d) R1 / R2 kgf
Answer
A
Question-10 A wheel of diameter 2 m is shown in Fig. 1.28 with axle at O. A force F = 2 N is applied at B in the direction shown in figure. Calculate the moment of force about (i) the centre O, and (ii) the point A.
A wheel of diameter 2 m is shown in Fig. 1.28 with axle at O. A force F = 2 N is applied at B in the direction shown in figure. Calculate the moment of force about (i) the centre O, and (ii) the point A.
(a) 2 Nm (clockwise), 4 Nm (anticlockwise)
(b) 2 Nm (anticlockwise), 4 Nm (clockwise)
(c) 2 Nm (anticlockwise), 4 Nm (anticlockwise)
(d) 2 Nm (clockwise), 4 Nm (clockwise)
Answer
D
Question 11
A body is acted upon by two unequal forces in opposite directions, but not in same line. The effect is that
(a) The body will have only the rotational motion
(b) The body will have only the translational motion
(c) The body will have neither the rotational motion nor the translational motion
(d) The body will have rotational as well as translational motion.
Answer
D
MCQ on Force Class 10 ICSE pdf are given here. You can easily download MCQ Questions for Class 10 ICSE Physics force pdf free of charge to score well in the exam. These books cover the whole ICSE syllabus as recommended by the Council. Preparing from these books will help in making concepts. Students can utilize this MCQ on Force Class 10 ICSE pdf for their board exams. Besides, this MCQ are great study material to prepare for competitive exams.
We hope the given MCQ Questions for Class 10 ICSE Physics Force Pdf with Answers free download will help you. If you have any issue in regard to Force Class 10 ICSE MCQ Questions Pdf. Drop a comment below and we will guide you as soon as possible.



