Students should refer to Previous Year Questions ICSE Class 10 Physics Force with solutions that have been prepared by expert teachers of ICSE Class 10 Physics. These questions and solutions are based on past year papers that have come in examinations of ICSE Class 10. Students should understand the type of questions asked and the solutions provided. Also refer to ICSE Class 10 Physics Solutions
ICSE Class 10 Physics Force Last Year Questions
Students should learn the important questions and answers given below for Chapter Force in Physics for ICSE Class 10. These board questions are expected to come in the upcoming exams. Students of ICSE Class 10th should go through the board exams questions and answers for ICSE Class 10 Physics which will help them to get more marks in exams.
Board Exam Questions Force ICSE Class 10 Physics
Previous Year Questions ICSE Class 10 Physics Force
Very Short Answer Type Questions
Question: What is the S.I unit of moment of force?
Answer: Newton × metre or N.m.
Question: A nut is opened by a wrench of length 20 cm. If the least force required is 2 N, find the moment of
force needed to loosen the nut.
Answer: Moment of force = Force × Moment arm
= 2X20X100
= 0.4 Nm
Question: What is the weight of a body placed at the centre of the earth?
Answer: Zero.
Short Answer Type Questions I
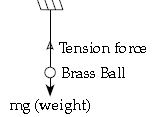
Question: A brass ball is hanging from a stiff cotton thread. Draw a neat labelled diagram showing the forces
acting on the brass ball and the cotton thread.
Answer: Weight of the ball acting vertically downwards and tension in the thread acting in opposite direction to
balance the weight.
Question: A uniform half metre rule balances horizontally on a knife edge at 29 cm mark when a weight of 20
gf is suspended form one end.
(i) Draw a diagram of the arrangement.
(ii) What is the weight of the half metre rule?
Answer: (i)
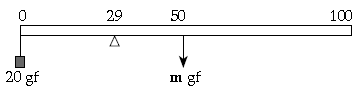
(ii) Applying principle of moments Anticlockwise moment = Clockwise moment
20 gf × (29 – 0) = m gf (50 – 29)
⇒ 20 × 29 = m × 21
⇒ 20×29/21
m = 27.6 gram
Question: Calculate the resultant torque from the following diagram :

Answer: Total torque acting in clockwise direction.
= -(30 × 4 + 50 × 5) = -(120 + 250) = -370 N-m.
Total torque acting in anticlockwise direction
= +(20 × 1 + 80 × 3) = +(20 + 240) = +260 N-m.
Torque due to 200 N will be zero because it is passing through point O about which troque is
calculated.
Net resultant torque = -370 + 260 = -110 N-m (Clockwise direction)
Question: A uniform metre scale is in equilibrium position. Calculate the mass of the ruler.

Answer:

Let, m be the mass of scale in gram
∴ Weight of scale = m gf
By principle of moment, we get
40 × (30 – 5) = m × (50 – 30)
[∴ centre of gravity of scale is 50 cm]
⇒ 40 × 25 = m × 20 ⇒ m = 50 g
Question: Name the factors affecting the turning effect of a body.
Answer: (i) Magnitude of the force applied.
(ii) Perpendicular distance of line of action of the force from the axis of rotation.
Question: (i) Define Equilibrium.
(ii) In a beam balance when the beam is balanced in horizontal position, it is in __ equilibrium.
Answer: (i) When a number of forces acting on a body produce no change in its state of rest or motion, the body is said to be in equilibrium.
(ii) Static.
Question: When a body is placed on a table top, it exerts a force equal to its weight downwards on the table
top but does not move or fall.
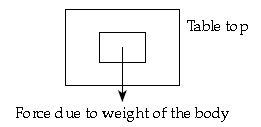
(i) Name the force exerted by the table top.
(ii) What is the direction of the force?
Answer: (i) Normal Reaction Force
(ii) Vertically Upwards
Question: A force is applied on (i) a non-rigid body and (ii) a rigid body. How does the effect of the force differ
in the above two cases?
Answer: (i) Dimension changes in the non-rigid body.
(ii) No change in dimensions.
Question: Two forces each of 5 N act vertically upwards and downwards respectively on the two ends of
a uniform metre rule which is placed at its midpoint as shown in the diagram. Determine the magnitude of the resultant moment of these forces about the midpoint.

Answer: Resultant moment of force = moment of couple
= F × Perpendicular distance between the forces
= 5 × 1
= 5 N.m
Question: One end of a spring is kept fixed while the other end in stretched by a force as shown in the diagram
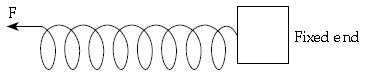
(i) Copy the diagram and mark on it the direction of the restoring force.
(ii) Name one instrument.
Answer:
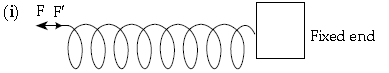
F′ is the restoring force
(ii) Spring balance.
Question: Give any two effects of a force on a non-rigid body.
Answer: The two effects of a force on a non-rigid body are :
(i) It can change the shape or size of the nonrigid body.
(ii) It can change the state of rest, motion or direction of a body.
Question: (i) Define 1 kgf.
(ii) How it is related to the S.I. unit of force?
Answer: (i) 1 kilogram force (1 kgf) : It is the amount of force with which earth attracts a mass of 1 kilogram
towards itself.
(ii) 1 kgf = 9.8 N
Question: A boy of mass 30 kg is sitting at a distance of 2 m from the middle of a see saw. Where should a boy
of mass 40 kg sit so as to balance the see saw?
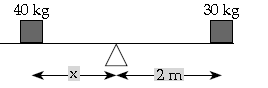
By principle of moments (for equilibrium) Anticlockwise moment = clockwise moment
⇒ 40 × x = 30 × 2
Question: (i) What is meant by the term ‘moment of force’?
(ii) If moment of force is assigned a negative sign will the turning tendency of the force be
clockwise or anti-clockwise?
Answer: (i) Moment of force : It is equal to the product of the magnitude of the force and the
perpendicular distance of the line of action from the axis of rotation.
(ii) Negative sign of moment of force depicts the clockwise turning tendency.
Question; (i) Define one Newton.
(ii) Write the relation between S.I. unit and C.G.S. unit of force.
Answer: (i) One Newton : It is the amount of force acting on a body of mass 1 kg which produces an acceleration of 1 m/s2 in it.
(ii) 1 newton = 105 dynes.
Question: A uniform meter scale can be balanced at the 70.5 cm mark when a mass of 0.05 kg is hung from
the 94 cm mark.
(i) Draw a diagram of the arrangement.
(ii) Find the mass of the meter scale.
Answer:
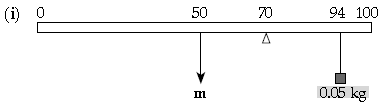
(ii) The centre of gravity of scale lies at 50 cm mark.
Anticlockwise moment = clockwise moment.
m × (70 – 50) = 0.05×(94 – 70)
m × 20 = 0.05 × 24
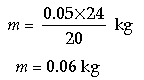
Question: A man can open a nut by applying a force of 150 N by using a lever handle of length 0.4 m. What should be the length of the handle, if he is able to open it by applying a force of 60 N?
Answer: Moment of force required to open the nut is given by
T = 150 × 0.4 Nm
= 60 Nm.
Now force to be applied = 60 N
Let the length of handle = x m
⇒ 60 Nm = 60 × x Nm
⇒ x = 1 m.
Question: A body of mass 1.50 kg is dropped from the 2nd floor of a building which is at a height of
12 m. What is the force acting on it during its fall?
(g = 9.80 m/s2)
Answer: Force acting during its free fall is given by
F = mg
= 1.50 × 9.8 N
= 14.7 N
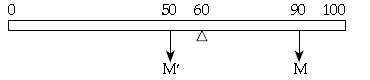
Question: A uniform metre scale is kept in equilibrium when supported at the 60 cm mark and a mass M
is suspended from the 90 cm mark as shown in the figure. State with reasons, whether the weight of
the scale is greater than, less than or equal to the weight of mass M.
Answer:
Let the mass of the scale = M′
Applying principle of moments Anti-clockwise moment = clockwise moment
⇒ M′ × (60 – 50) = M×(90 – 60)
⇒ M′ × 10 = M × 30
⇒ M′ = 3 M
Hence mass the scale is more than the mass suspended.
Question: Name the gravitational units of force in
(i) C.G.S. units and
(ii) S.I. units
Answer: (i) gram-force (gf)
(ii) kilogram-force (kgf)
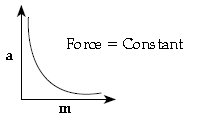
Question: Draw a graph showing the relation between acceleration and mass for a constant force
Answer:
Question: Draw a graph showing the relation between acceleration and force for a constant mass. What does the slope of this graph depicts?
Answer:
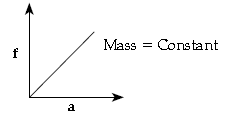
The slope of ‘force-acceleration’ graph depicts the mass of the body.
Question: Draw a graph showing the relation between force and mass. What does the slope of this graph depicts?
Answer:
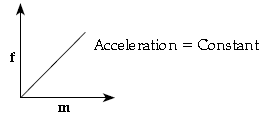
The slope of ‘force-mass’ graph depicts the acceleration of that body.
Question: A force ‘F’ acts on a body such that line of action of force passes through the point of rotation of the
body. Find the magnitude of moment of force.
Answer: Here, force = F
Perpendicular distance of force from point of rotation = 0
Moment of force = F × 0
= 0.
Question: A force of 3.5 N, with the length of its moment arm being 6 m, rotates the body in anti-clockwise direction. Find the torque.
Answer: Force = 3.5 N
Distance = 6 m
Moment of force = 3.5 × 6
= 21 Nm.
Question: If a body is acted upon by two torques of 9 Nm anti-clockwise and 15 Nm clockwise. What is the resultant torque on the body?
Answer: Anti-clockwise torque = +9 Nm
Clockwise toque = -15 Nm
Net Torque = +9 Nm – 15 Nm = -6 Nm
Negative sign depicts that net torque is in clockwise direction.
Question: The man weighing 60 kgf, is sitting at a distance of 2.5 m from the centre of a see-saw. Where must
a boy weighing 50 kgf, sit on the opposite side of the centre so as to keep the see-saw balanced?
Answer:
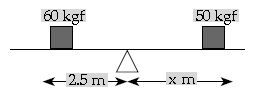
Applying principle of moments
60 kg × 2.5 m = 50 kg × x m
Anti-clockwise moment = Clockwise moment
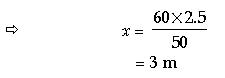
Question: A couple is formed by two equal and opposite forces of 40 N each. The distance between line of action of forces is 5 m. Calculate the moment of couple. If the point, about which rotation takes place, is shifted, will there be any change in moment of couple?
Answer: Moment of couple = 40 × 5
= 200 Nm
No if the point about which rotation takes place, shifts, there is no change in moment of couple
Short Answer Type Questions II
Question: (i) Define the term momentum.
(ii) How is force related to the momentum of a body?
(iii) State the condition when the change in momentum of a body depends only on the change in its velocity.
Answer: (i) Momentum of a body is the quantity of motion contained in a body. It is given by the product of mass and the velocity. It is a vector quantity.
Its S.I. unit is Kg ms-1.
P = mv
Where m is the mass of the body and V is it’s velocity
(ii) The rate of change of momentum of a body is directly proportional to the force applied. i.e.,
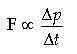
(iii) The change in momentum of a body depends only on the change in its velocity when, mass remains constant. If the change in velocity is small, mass almost remains same.
Question: A uniform meter scale is put on a knife edge at 40 cm mark. It is found that this scale gets balanced when
a weight of 20 gf is suspended from the 20 cm mark. Find the weight of meter scale.
Answer:
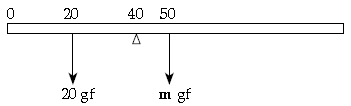
Since the meter scale is uniform hence its centre of gravity acts at 50 cm mark. Applying principle of moments
Anti-clockwise moment = Clockwise moment
⇒ 20 × (40-20) = m × (50 – 40)
⇒ 20 × 20 = m × 10
⇒ m = 400/10
⇒ m = 40 g
Wight of meter scale = 40 gf
Long Answer Type Questions
Question: A meter scale is kept on a knife edge at 50 cm mark. A weight of 20 gf is hanging at 80 cm mark and a weight of 50 gf is hanging at 60 cm mark. Where should a weight of 55 gf be placed to make the meter scale balanced?
Answer:

Let the position of 55 gf weight be x cm on meter scale. It is clear from the figure that 55 gf weight must be
placed on the side other than the side containing 50 gf and 20 gf weights.
Applying principle of moments Anti-clockwise moment = clockwise moment
55 × (50 – x) = 50 × (60 – 50) + 20 × (80 – 50)
⇒ 55 × (50 – x) = 50 × 10 + 20 × 30
⇒ 55 × (50 – x) = 500 + 600
⇒ 50 – x = 1100/55
⇒ 50 – x = 20
⇒ x = 50 – 20
⇒ x = 30 cm
Hence the weight of 55 gf must be placed at 30 cm mark.
Centre of Gravity and Uniform Circular Motion
Very Short Answer Type Questions
Question: How does uniform circular motion differ from uniform linear motion?
Answer: In uniform circular motion, the direction of motion keeps on changing continuously while in uniform linear motion, the direction of motion remains same.
Question: On what factors does the position of the centre of gravity of a body depend?
Answer: Shape of body/distribution of mass of body.
Question: What name is given to the acceleration experienced by a particle in uniform circular motion.
Answer: Centripetal acceleration.
Question: Can a particle move in a circular path even when no centripetal force is acting on it?
Answer: No.
Question: A stone is tied to a string and whirled around in a circular path. What is the source of centripetal force acting on it?
Answer: Tension in the string.
Question: What provides the necessary centripetal force for the motion of moon around the earth?
Answer: The force of gravitational attraction exerted by the earth on moon.
Question: What is the position of the centre of gravity of a triangular lamina?
Answer: At centroid (intersection point of medians).
Short Answer type Questions I
Question: Where is the centre of gravity of the following objects situated?
1. Ring
2. Rhombus
3. Scalene triangle
4. Cylinder.
Answer: 1. Ring—at the centre of ring
2. Rhombus—point of intersection of diagonals
3. Scalene triangle—point of intersection of medians
4. Cylinder—at the mid-point on the axis of cylinder
Question: Explain the motion of a planet around the sun in a circular path.
Answer: A planet moves around the sun in a nearly circular path for which the gravitational force of attraction on the planet by the sun provides the centripetal force.
Question: Is it possible to have an accelerated motion with a constant speed? Explain.
Answer: Yes, the velocity of a particle in a uniform circular motion is variable or the circular motion is
accelerated even though the speed of particle is uniform
Question: Where does the position of centre of gravity lie for
(i) A circular lamina
(ii) A triangular lamina?
Answer: (i) At the centre of circle
(ii) At intersection point of medians i.e., centroid.
Question: When a person standing on one foot, bends forward and his second leg automatically goes
backwards. Why?
Answer: The second leg goes backwards so as to keep the centre of gravity over the supporting foot.
Question: Where is the centre of gravity of
(i) A circular ring
(ii) Rhombus
Answer: (i) At the centre of ring.
(iii) At the intersection point of diagonals.
Question: State the direction of :
(i) Centripetal force
(ii) Centrifugal force
Answer (i) Radially inwards towards the centre.
(ii) radially outwards away from the centre.
Short Answer Type Questions II
Question: A stone of mass ‘m’ is rotated in a circular path with a uniform speed by tying a string with the help of
your hand. Answer the following questions :
(i) Is the stone moving with a uniform or variable speed?
(ii) Is the stone moving with a uniform acceleration? In which direction does the acceleration act?
(iii) What kind of force acts on the hand and state its direction?
Answer: (i) Uniform speed.
(ii) Yes, radially inwards.
(iii) Centrifugal force acting radially outward.
Question: With reference to their direction of action, how does a centripetal force differ from a centrifugal force?
Answer: With reference to their direction of action, centripetal force is directed towards the centre of circle where as centrifugal force is opposite to centripetal force. (i.e., radially outward)
Question: (i) Which of the following remains constant in uniform circular motion, speed or velocity or both?
(ii) Name the force required for uniform circular motion. State its direction.
Answer: (i) Speed remains constant in uniform circular motion.
(ii) Centripetal force is required for uniform circular motion. Centripetal force is directed towards the centre.



