Sample Paper ICSE Class 10 Mathematics Set A

Students can refer to the following Sample Paper ICSE Class 10 Mathematics Set A with Answers provided below based on the latest syllabus and examination guidelines issued for ICSE Mathematics. All specimen papers have been prepared covering all chapters given in ICSE Mathematics book for Class 10. You should also refer to ICSE Class 10 Mathematics Solutions.

Sample Paper ICSE Class 10 Mathematics Set A with Answers

Class: TEN (X) Full Marks:80
Subject: MATHEMATICS Pass Marks:

(Candidates are allowed additional 15minutes for only reading the paper. They must not start writing during this time
SECTION-A (40 Marks)
Attempt all questions from this section

Question -1 a. Find x, y if

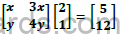
b. Harish deposited Rs. 150 per month in a bank for 8 months under the Recurring Deposit Scheme. What will be the maturity value of his deposits if the rate of interest is 8% per annum and interest is calculated at the end of every month?

c. Cards bearing numbers 2,4,6,8,10,12,14,16,18 & 20 are kept in a bag. A card is drawn at random from the bag. Find the probability of getting a card which is :
i. a prime number
ii. a number divisible by 4
iii. a number that is a multiple of 6
iv. an odd number

Question -2
a. The capacity and the base area of a right circular vessel are 9856 𝒄𝒎𝟑 and 616 𝒄𝒎^𝟐respectively. Find the curved surface area of the vessel. [3]
b. Determine the value of k for which 𝒌^𝟐+𝟒𝒌+𝟖,𝟐 𝒌^𝟐+𝟑𝒌+𝟔,𝟑 𝒌^𝟐+𝟒𝒌+𝟒 are in A.P. [3]
c. PQRS is a cyclic quadrilateral. Given ∠QPS=73˚ , ∠PQS=55˚ & ∠PSR=82˚, calculate :
i. ∠QRS
ii. ∠RQS
iii. ∠PRQ [4]

Question -3
a. Find ‘a’ if the two polynomials 𝒂𝒙^𝟑+𝟑𝒙^𝟐−𝟗 & 2𝒙^𝟑+𝟒𝒙+𝒂 leave the same remainder when divided by x+3.

b. Prove that √(𝟏−𝒄𝒐𝒔𝑨/𝟏+𝒄𝒐𝒔𝑨)=(𝒔𝒊𝒏𝑨/𝟏+𝒄𝒐𝒔𝑨)

c. Find the value of p , if the mean of the following distribution is 20.

Question -4
a. Solve the following inequation & represent the solution set on the number line :
𝟒𝒙−𝟏𝟗 ˂𝟑𝒙/𝟓−𝟐 ≤ −𝟐/𝟓+𝒙, 𝒙ε𝑹 [3]
b. Find the equation of the line which passes through (2,7) & whose y-intercept is 3. [3]
c. Find the value of ‘m’ if (𝒎−𝟐)𝒙^𝟐– (𝟓+𝒎)𝒙+𝟏𝟔=𝟎 has equal roots.

SECTION-B (40 Marks)
Attempt any four questions from this section

Question -5
a. The sum of n natural numbers is 𝟓𝒏^𝟐+𝟒𝒏. Find its 8th term. [3]

b. The marked price of an article is Rs 6000. A wholesaler sells it to a dealer at 20% discount . The dealer further sells the article to a customer at a discount of 10% on the marked price. If the rate of GST at each stage is 18% , find the amount of tax(under GST) paid by the dealer to the Government. [3]

c. Using a graph paper plot the points A(6,4) & B(0,4).
i) Reflect A & B in the origin to get the images A’ & B’
ii) Write the coordinates of A’ & B’
iii) State the geometrical name for the figure ABA’B’
iv) Find its perimeter.

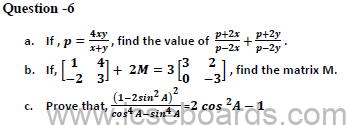
Question -7
a. Solve 𝟒(𝟐𝒙−𝟑)^𝟐−(𝟐𝒙−𝟑)−𝟏𝟒=𝟎. [3]

b. ABC is a triangle right angled at B. D & E are two points on AC & BC respectively and AB & DE are perpendiculars to BC.
i) Prove that, Δ ABC ~Δ DEC
ii) If, AB= 6 cm, DE= 4 cm & AC= 15 cm. Calculate CD. [3]

c. The line joining P(-4,5) & Q(3,2) intersects the y-axis at point R. PM & QN are perpendiculars from P & Q on the x-axis. Find:
i) the ratio PR : RQ
ii) the coordinates of R
iii) the area the quadrilateral PMNQ.

Question -8
a. Three consecutive natural numbers are such that the square of the middle number exceeds the difference of the squares of the other two by 60. Assume the middle number to be x & form a quadratic equation satisfying the above statement. Hence find three numbers. [3]

b. Find the mean of the following distribution in Short Cut Method.

c. In quadrilateral ABCD , ∠D=90˚, BC=38 cm & DC= 25 cm. A circle is inscribed in this quadrilateral which touches AB at point Q such that QB=27 cm. Find the radius of the circle.

Question -9
a. Mira has a recurring deposit account in a bank. She deposits Rs. 2500 per month for 2 years . If she gets Rs. 66250 at the time of maturity , find :
i) the interest paid by the bank
ii) the rate of interest. [3]

b. In Δ ABC , ∠ABC=∠DAC, AB= 8 cm, AC=4 cm, & AD= 5 cm.
i) Prove that ΔACD is similar to ΔBCA
ii) Find BC & CD
iii) Find area of ΔACD : area of ΔABC [3]

c. Find the volume of the largest cylinder formed when a rectangular piece of paper 22 cm by 15 cm is rolled along its longer side.

Question -10
a. If (x-2) is a factor of 𝟐𝒙^𝟑−𝒙^𝟐−𝒑^𝒙−𝟐,
i) find the value of p
ii) with the value of p , factorise the above expression completely. [3]

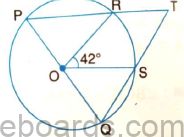
b. In the given figure , PQ is the diameter of the circle whose centre is O. Given ∠ROS=42˚ , calculate ∠RTS.

c. A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60˚. When he was 40 km away from the bank he finds that the angle of elevation to be 30˚.
i) Find the height of the tree.
ii) Find the width of the river, correct to two decimal places.

Question -11
a. Five years ago a woman’s age was the square of her son’s age. Ten years hence her age will be twice that of her son’s age. Find:
i) the age of the son 5 years ago
ii) the present age of the woman.

b. A survey regarding height (in cm) of 60 boys belonging to class X of a school was conducted. The following data was recorded:

Taking 2 cm= height of 10 cm along one axis & 2cm= 10 boys along the other axis, draw an ogive of the above distribution. Use graph to estimate the following:
i) the median
ii) lower quartile
iii) if above 158 cm is considered as the tall boys of the class, find the number of boys in the class who are tall.