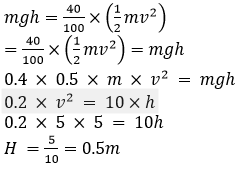Exercise 2(A)
Question 1. Define work. Is work a scalar or a vector?
Solution:
Only when a body moves as a result of a force being applied to it is stated to have been done. It’s a scalar amount.
Question 2. How is the work done by a force measured when:
1. the force is in direction of displacement
2. the force is in the direction of displacement?
Solution:
(1) Work is completed when force is in the direction of displacement, W = F S.
(2) Cos0˚ = 1 when force is acting in the direction of displacement with angle = 0.
As a result, the work performed by a force is W = F*S when measured in the direction of displacement.
The job completed is extensive and productive.
Question 3. A force F acts on a body and displaces it by a distance S in a direction at an angle θ with the direction of force.
1. Write the expression for the work done by the force.
2. What should be the angle between force and displacement so that the work done is (i) zero, (ii) maximum?
Solution:
(1) When force is at an angle to the direction of displacement, then work done, W = F S cos θ
(2) i) For zero work done, the angle between force and displacement should be 90o as cos 90o=0
W = FS cos 90o = FS x 0 = 0
(ii) For maximum work done, the angle between force and displacement should be 0o as
cos0o = 1
Hence, W = FS cos0o=FS
Question 4. A body is acted upon by a force. State two condition when the work done is zero.
Solution:
Two conditions when the work done is zero are:
When there is no displacement (S=0) and when the displacement is perpendicular to the direction of the force (=90o), respectively, the work done is zero.
Question 5. State the condition when the work done by a force is
1. Positive
2. Negative
Explain with the help of an example
Solution:
(1) Positive work is done if the body is moved in the direction of the force.
Thus, W= F x S.
When a coolie raises a load against gravity, for instance, he is working on the load. Both the displacement and the force applied by the coolie (=mg) are in the upward direction
(2) Work is not done if the body is moved in the direction that is opposed to the force.
Thus, W = -F × S.
For instance, when a body travels over a surface, the force of friction between the body and the surface acts in the direction opposite to the body’s motion, producing negative work.
Question 6. A body is moved in a direction opposite to the direction of force acting on it. State whether the work is done by the force or work is done against the force
Solution:
Work is done in opposition to the force.
Question 7. When a body moves in a circular path, how much work is done by the body? Give reason.
Solution:
When a body moves around a circle, no work is done since the force acting on the body is centripetal, or directed toward the center of the circle. However, the displacement is always along the tangent to the circle, or normal to the direction of the force
Question 8. A satellite revolves around the earth in a circular orbit. What is the work done by the force of gravity? Give reason.
Solution:
Since the force of gravity operating on the satellite is normal to the movement of the satellite, the work done by the force of gravity (which generates the centripetal force) is zero.
Question 9. In which of the following cases, is work being done?
1. A man pushing a wall.
2. a coolie standing with a load of 12 kgf on his head.
3. A boy climbing up a staircase.
Solution:
Work is done only in case of a boy climbing up a stair case.
Question 10. A coolie X Carrying a load on his head climbs up a slope and another coolie Y carrying the identical load on his head move the same distance on a frictionless horizontal platform. Who does more work? Explain the reason.
Solution:
Energy is the capacity to do work and work done is equal to energy spent. Coolie X carrying a load up a slope will do more work as this works involve a change in potential energy, kinetic energy and loss of energy due to friction. Work done in carrying the load in horizontal frictionless surface does not involve change in potential energy and work done by the friction is also zero
Question 11. The work done by a fielder when he takes a catch in a cricket match, is negative Explain.
Solution:
Force applied by the fielder on the ball is in opposite direction of displacement of ball. So, work done by the fielder on the ball is negative.
Question 12. Give an example when work done by the force of gravity acting on a body is zero even though the body gets displaces from its initial position.
Solution:
When a coolie carries a load while moving on a ground, the displacement is in the horizontal direction while the force of gravity acts vertically downward. So the work done by the force of gravity is zero.
Question 13. What are the S.I. and C.G.S units of work? How are they related? Establish the relationship.
Solution:
S.I unit of work is Joule. C.G.S unit of work is erg.
Relation between joule and erg : 1 joule = 1N x 1m
But 1N = 105 dyne And 1m=100 cm = 102 cm
Hence, 1 joule = 105dyne × 102cm
= 107dyne × cm =107erg
Thus, 1 Joule = 107 erg
Question 14. State and define the S.I. unit of work.
Solution:
S.I unit of work is Joule. 1 joule of work is said to be done when a force of 1 newton displaces a body through a distance of 1 meter in its own direction.
Question 15. Express joule in terms of erg
Solution:
Relation between joule and erg : 1 joule = 1N × 1m But 1N = 105 dyne and 1m =100 cm = 102 cm Hence, 1 joule = 105 dyne × 102 cm = 107 dyne × cm = 107 erg
Thus, 1 Joule = 107 erg.
Question 16. A body of mass m falls down through a height h. Obtain an expression for the work done by the force of gravity.
Solution:
Allow a mass m body to descend down a vertical height h directly or through an inclined surface, such as a hill, slope, or stairway. The displacement in the direction of the force (i.e., vertical) is S = h, and the force of gravity acting on the body is F = mg acting vertically downwards. As a result, W = FS = mgh represents the work done by the force of gravity.
Question 17. A boy of mass m climbs up a staircase of vertical height h.
1. What is the work done by the boy against the force of gravity?
2. What would have been the work done if he uses a lift in climbing the same vertical height?
Solution:
Give a boy with mass m the option of using a lift or a staircase to ascend a vertical height h. The youngster is subject to a vertical downward acting force of gravity called F = mg, and the displacement in the direction vertically opposed to that force is called S = h. As a result, either the boy does the work W = mgh against the force of gravity, or the force of gravity does the work W = mgh on the boy.
Question 18. Define the term energy and state its S.I. unit.
Solution:
The ability of a body to perform labor is its energy. Joule is its SI unit (J)
Question 19. What physical quantity does the electron volt (eV) measure? How is it related to the S.I. unit of that quality?
Solution:
Electron Volt (eV) measures the energy of atomic particles.
1eV = 1.6 × 10-19J
Question 20. Complete the following sentence:
1. 1 J = Calorie
2. 1 kWh = ______ J.
Solution:
1. 1 J = 0.24 calorie
2. 1 kWh = 3.6 × 106 J
Question 21. Name the physical quantity which is measured in calorie. How is it related to the S.I. unit of the quality?
Solution:
Calorie measures heat energy.
1calorie = 4.18 J
Question 22. Define a kilowatt hour. How is it related to joule?
Solution:
A source of power expends 1 kWh of energy (or does 1 kWh of effort). 1kW in 1 h.
1kWh = 3.6 x 106J
Question 23. Define the term power. State its S.I. unit.
Solution:
Electric power is the rate, per unit time, at which electrical energy is transferred by an electric circuit. The SI unit of power is the watt, one joule per second
Question 24. State two factors on which power spent by a source depends. Explain your answer with examples.
Solution:
The quantity of work done by a source and the length of time it takes to do that task are the two variables that determine how much power is expended by that source.
Example: If a coolie A lifts a weight to the roof of a bus in one minute and a coolie B lifts the same load in two minutes, the work performed by both coolies is same, but the power used by coolie A is twice as much as the power used by coolie B since the coolie A works more quickly.
Question 25. Differentiate between work and power.
Solution:
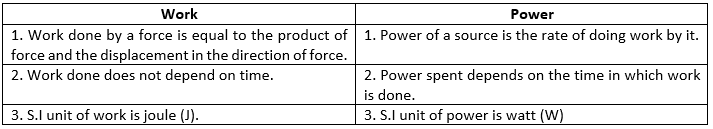
Question 26. Differentiate between energy and power.
Solution:
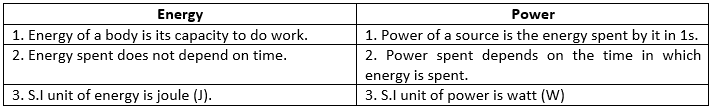
Question 27. State and define the S.I. unit of power.
Solution:
Watt is the SI unit of power (W).
The power used is defined as 1 watt if 1 joule of work is completed in 1 second.
Question 28. (1) Name the physical quantity measured in terms of horse power.
(2) How is horse power related to the S. I. unit of power?
Solution:
1. Power is measured in terms of horse power.
2. 1 horse power =746 watt
Question 29. Differentiate between watt and watt hour.
Solution:
Watt (W) is the unit of power, while watt hour (Wh) is the unit of work, since power × time = work
Question 30. Name the quality which is measured in:-
1. kwh
2. kw
3. Wh
4. eV
Solution:
1. Energy is measured in kWh.
2. Power is measure in kW.
3. Power is measure in Wh.
4. Energy is measured in eV.
Question 31. Is it possible that no transfer of energy may take place even when a force is applied to a body?
Solution:
Yes, there is no energy transfer when force and displacement are normal.
Exercise -2(A)
Multiple Choice Type:-
Question 1. One horse power is equal to:
a) 1000 W
b) 500 W
c) 764 W
d) 746 W
Solution: d) 746 W
Question 2. kWh is the unit of:
a) Power
b) Force
c) Energy
d) None of these
Solution: c) energy.
Question 1. A body, when acted upon by a force of 10 kgf, gets displaced by 0.5 m. Calculate the work done by the force, when the displacement is (i) in the direction of force, (ii) at an angle of 60° with the force, and (iii) normal to the force. (g = 10 N kg-1)
Solution:
Force acting on the body = 10 kgf = 10 × 10 N = 100 N
Displacement, S = 0.5 m
Work done = force x displacement in the direction of force
(i) W = F × S
W = 100 × 0.5= 50 J
(ii) Work = force x displacement in the direction of force
W = F × S cosθ
W = 100 × 0.5 cos60o
W = 100 × 0.5 × 0.5(cos 60o = 0.5)
W = 25 J
(iii) Normal to the force:
Work = force x displacement in the direction of force
W = F × S cosθ
W = 100 × 0.5 cos90o
W = 100 × 0.5 × 0 = 0 J(cos90o = 0)
Question 2. A boy of mass kg runs upstairs and reaches the 8m high floor in 5s Calculate:
the force of gravity acting on the boy.
(1) the work done by him against gravity.
(2) the power spent by boy.
(Take g = 10 m s-2)
Solution:
Mass of boy = 40 kg Vertical height moved, h = 8m Time taken, t = 5s.
1. Force of gravity on the boy F = mg = 40 × 10 = 400N
2. While climbing, the boy has to do work against the force of gravity. Work done by the boy in climbing= Force × distance moved in the direction of force,
W = F × S
W = 400 × 8
W = 3200J
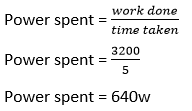
Question 3. A man spends 6.4 KJ energy in displacing a body by 64 m in the direction in which he applies force, in 2.5 s Calculate:
1. the force applied
2. the power spent (in H.P) by the man.
Solution:
1. Work done by man = 6.4kJ
Distance moved, S = 64m
Work done by the man= Force x distance moved in direction of force
Work, W = F × S
6.4 × 103 = F × 64
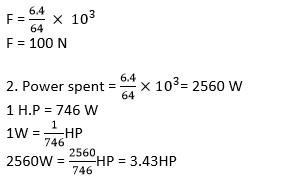
Question 4. A weight lifter a load of 200 kgf to a height of 2.5 m in 5 s. Calculate: (i) the work done, and (ii) the power developed by him. Take g = 10 N kg-1
Solution:
Force= mg = 200 × 10 = 2000 N
Distance, S = 2.5m
Time , t = 5 s
(i) Work done, W= F S
W = 2000 × 2.5m = 5000J
Power developed = work done time taken= 5000J5s=1000W
Question 5. A machine raises a load of 750 N through a height of 16 m in 5 s. Calculate:
(1) energy spent by machine,
(2) power at which the machine works.
Solution:
1. Energy spent by machine or work done = F S
Work, W = 750 × 16 = 12000J
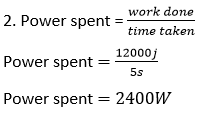
Question 6. An electric heater of power 3 KW is used for 10 h. How much energy does it consume? Express your answer in (i) kWh, (ii) joule.
Solution:
Energy consumed = power x time
1) Energy = 3 kW × 10 h = 30 kWh
2) 1 kilowatt hour (kWh) = 3.6 x 106J
30kWh = 30 × 3.6 ×106
= 1.08 ×108
Question 7. A water pump raises 50 litres of water through a height of 25 m in 5 s. Calculate the power which the pump supplies.
(Take g = 10 N kg-1 and density of water = 1000 kgm-3)
Solution:
Volume of water = 50L=50×10-3 m-3
Density of water = 1000 kgm-3
Mass of water = Volume of water × density of water
= 50×10-3 × 1000 = 50kg
Work done in raising 50kg water to a height of 25m against the force of gravity is
W = mg × h = mgh
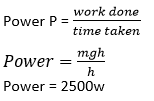
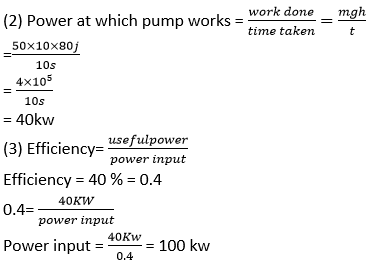
Question 8. A pump is used to lift 500 kg of water from a depth of 80 m in 10s. calculate:
(1) The work done by the pump
(2) The power at which the pump works
(3) The power rating of the pump if its efficiency is 40%. (Take g= 10ms-2).
Solution:
(1) W = mg × h = mgh
W = 500×10×80
= 4×105J
Question 9. An ox can apply a maximum force of 1000 N. It is taking part in a cart race and is able to pull the cart at a constant speed of 30 MS-1 while making its best effort. Calculate the power developed by the ox.
Solution:
It is given that,
Force = 1000N,
Velocity = 30m/s
Power, P = force × velocity
P = 1000 × 30 = 30,000W = 30kW
Question 10. If the power of a motor is 40 KW, at what speed can it raise a load of 20,000 N?
Solution:
Power = 40KW
Force = 20,000N
Power = force × velocity

Question 11. Rajan exerts a force of 150 N in pulling a cart at a constant speed of 10 m/s. Calculate the power exerted.
Solution:
It is given that, Force = 150 N
Velocity = 10 ms-1
Power = F x v
Power = 150 N x 10ms-1
Power = 1500 W
Question 12. A boy weighing 350 N runs up a flight of 30 steps, each 20 cm high in 1 minute, Calculate:
(i) the work done and
(ii) power spent.
Solution:
Total distance covered in 30 steps , S= 30 x 20 cm = 600 cm = 6 m
Work done by the boy in climbing = Force x distance moved in direction of force
Work, W= F x S= 350 x 6 = 2100J
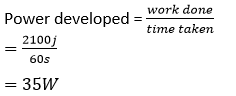
Question 13. It takes 20 s for a person A of mass 50 kg to climb up the stairs, while another person B does the same in 15 s.
1. Compare the Work done.
2. Compare the power developed by persons A and B.
Solution:
1.) Comparison of the word done:-
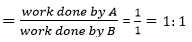
2. A takes 20 s to climb the stairs while B takes 15 s, to do the same. Hence B does work at a much faster rate than A; more power is spent by B.
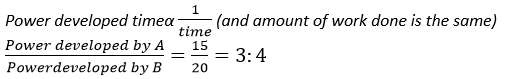
Question 14. A boy of weight 40 kgf climbs up the 15 steps, each 15 cm high in 10 s and a girl of weight 20 kg does the same in 5 s. Compare
1. the work done
2. Take g = 10 N kg-1.
Solution:
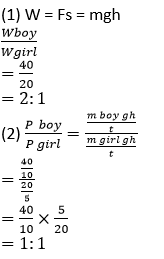
Question 15. .(1) A man raises a box of mass 50kg to a height of 2m in 20s, while another man raises the same box to the same height in 50s. Compare:
(i) the work done, and
(ii) the power developed by them.
(2) (i) the work done, and
(ii) the power developed by each man. Take g = 10N kg-1.
Solution:
(1) (i) Work done to raise the block of mass 50kg is same for both.
(ii) Power= Work done/time. As the time taken by the first man is less therefore power developed is more.
(2) (i) Work done = 50 × 10 × 2 = 1000 J
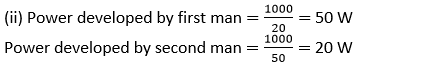
Question 16. A boy takes 3 minutes to lift a 20 litre water bucket from a 20 m deep well, while his father does it in 2 minutes. compare :
(1) (i) the work, and
(ii) power developed by them.
(2) Take density of water = 103 kg m-3 and g = 9.8 N kg-1.
Solution:
1. (i) Work done is same for both as both the people carry the same weight of water to the same height.
(ii) Power developed is
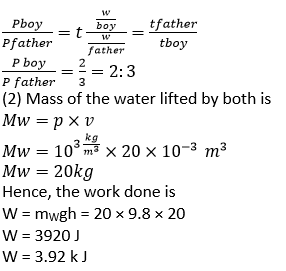
Exercise 2(B)
Question 1. What are the two forms of mechanical energy?
Solution:
The first is kinetic energy, the second is potential energy.
Question 2. Name the forms of energy which a wound-up watch spring possesses.
Solution:
A wound watch spring possesses elastic potential energy.
Question 3. Name the type of energy (kinetic energy K or potential energy U) possessed in the given cases:
1. A moving cricket ball
2. A compressed spring
3. A moving bus
4. A stretched wire
5. An arrow shot out of a bow
6. A piece of stone places on the roof.
Solution:
(1) Kinetic energy (K)
(2) Potential energy (U)
(3) Kinetic energy (K)
(4) Kinetic energy (K)
(5) Kinetic energy (K)
(6) Potential energy (U)
Question 4. Define the term
1. Potential energy of a body.
2. State different forms of potential energy and give one example of each.
Solution:
(1) Potential energy: Potential energy is the energy that a body possesses due to its particular location (or altered shape).
(2) The following list of P.E. types is provided:
(i) Gravitational potential energy: A body’s gravitational potential energy is the energy it possesses as a result of its position in relation to the center of the Earth.
Example: Because of its elevated height, a stone at a height has gravitational potential energy.
(ii) Elastic potential energy: A body’s elastic potential energy is the potential energy it has when it is deformed owing to a change in its configuration.
Example: Because of its compressed condition, a spring has elastic potential energy.
Question 5. Name the form of energy which a body may possess even when it is not in motion. Give an example to support your answer.
Solution:
The body always has potential energy, even when it is not moving. As an illustration, a stone lifted at a height has gravitational potential energy because of its elevated location.
Question 6. What is meant by the gravitational potential energy? Derive expression for it.
Solution:
The potential energy that a body has because of its location in relation to the center of the earth is known as gravitational potential energy.
The amount of labor required to pull a body up to a certain height above the ground is used to calculate the gravitational potential energy for that body.
Lift a mass m body vertically up from the ground to a height h. The force of gravity (=mg) acting vertically downward on the body must be equal to the minimum upward force F necessary to lift the body (without acceleration). The effort required to raise a body to a height h is given by W = force of gravity (mg) displacement (h) = mgh. The effort required to raise a body to a height h is given by W= force of gravity (mg) displacement (h) = mgh.
Question 7. Write an expression for the potential energy of a body of mass m places at a height h above the earth’s surface
Solution:
The effort required to raise a body to a height h is given by W= force of gravity (mg) displacement (h) = mgh.
When the body is at a height h, this work is stored in it as gravitational potential energy.
U = mgh, or the gravitational potential energy.
Question 8. What do you understand by the kinetic energy of a body?
Solution:
Kinetic energy is described as being within a moving body. Kinetic energy is the power that a body possesses as a result of its motion.
Question 9. A body of mass m is moving with a velocity v.
1. Write the expression for its kinetic energy.
2. Show that the quantity 2K/v2 has the unit of mass, where K is the kinetic energy of the body.
Solution:
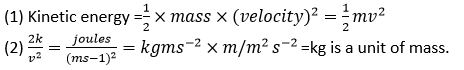
Question 10. State the work energy theorem.
Solution:
The work-energy theorem states that the work a force exerts on a moving body is equal to the rise in kinetic energy.
Question 11. A body of mass m is moving with a uniform velocity u. A force is applied on the body due to which its velocity changes from u to v. How much work is being done by the force.
Solution:
Body of mass m is moving with a uniform velocity u. A force is applied on the body due to which its velocity changes from u to v and produces an acceleration a in moving a distance S. Then, Work done by the force = force × displacement
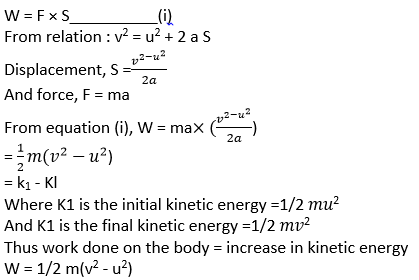
Question 12. A light mass and a heavy mass have equal momentum. Which will have more kinetic energy?
(Hint : Kinetic energy K = P2/2m where P is the momentum)
Solution:
Kinetic energy, k= p2/2 m where p is the momentum.
Both the masses have same momentum p. The kinetic energy, K is inversely proportional to mass of the body.
Hence light mass body has more kinetic energy because smaller the mass, larger is the kinetic energy.
Question 13. Two bodies A and B of masses m and M (M≫ m) have same kinetic energy. Which body will have more momentum?
Solution:
Kinetic energy is related to momentum and mass as
p = √2mk
As the kinetic energy of both bodies are same, momentum is directly proportional to square root of mass.
Now, mass of body B is greater than that of body A.
Hence, body B will have more momentum than body A
Question 14. Name the three forms of kinetic energy and give on example of each.
Solution:
These are the three types of kinetic energy:
(i) A body falling freely is an example of I translational kinetic energy; a spinning top is an example of
(ii) Rotational kinetic energy.
(iii) Atoms in a solid oscillating around their mean location is an example of vibrational kinetic energy
Question 15. Differentiate between the potential energy (U) and the kinetic energy (K)
Solution:

Question 16. Complete the following sentence:
1. The kinetic energy of a body is the energy by virtue of its………….
2. The potential energy of a body is the energy by virtue of its ……………….
Solution:
1. Motion.
2. Position.
Question 17. When an arrow is shot from a bow, it has kinetic energy in it. Explain briefly from where does it get its kinetic energy?
Solution:
When a bow’s string is pulled, some work is completed and is then stored as the elastic potential energy of the bow in its deformed condition. The potential energy of the bow transforms into the kinetic energy of the arrow when the string is released to shoot it, which causes it to move.
Question 18. A ball is placed on a compressed spring. What form of energy does the spring possess? On releasing the spring, the ball flies away. Give a reason.
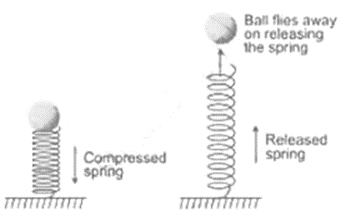
Solution:
Due to its compressed condition, the spring has elastic potential energy. When it is released, the spring’s potential energy transforms into kinetic energy, which moves a ball when it is put on it and causes it to fly away when it is not.
Question 19. A pebble is thrown up. It goes to a height and then comes back on the ground. State the different changes in form of energy during its motion.
Solution:
The pebble’s kinetic energy becomes potential energy when it is flung upward.
At the pinnacle of its motion, all of its kinetic energy is transformed into potential energy.
Potential energy undergoes partial conversion to kinetic energy as it descends, and complete conversion to kinetic energy occurs at the bottom.
Question 20. In what way does the temperature of water at the bottom of a waterfall differ from the temperature at the top? Explain the reason.
Solution:
The potential energy held in water at a height transforms into the kinetic energy of water during the fall when it is dropped from a height. When water hits the ground, some of its kinetic energy transforms into heat energy, raising the water’s temperature.
Question 21. Name the form of energy in which potential energy can change
Solution:
Kinetic energy
Question 22. Name the form of mechanical energy, which is put to use.
Solution:
Kinetic energy
Question 23. Name six different forms of energy?
Solution:
The six different forms of energy are:
1. Solar energy
2. Heat energy
3. Light energy
4. Chemical or fuel energy
5. Hydro energy
6. Nuclear energy
Question 24. Energy can exist in several forms and may change from one form to another. For the following, state the energy changes that occur in:
1. the unwinding of a watch spring
2. a loaded truck when started and set in motion
3. a car going uphill
4. Photosynthesis in green leaves.
5. Charging of a battery.
6. respiration
7. burning of a match stick
8. explosion of crackers.
Solution:
(1) Potential energy of wound up spring converts into kinetic energy.
(2) Chemical energy of petrol or diesel converts into mechanical energy (kinetic energy)
(3) Kinetic energy to potential energy
(4) Light energy changes into chemical energy.
(5) Electrical energy changes into chemical energy.
(6) Chemical energy changes into heat energy.
(7) Chemical energy changes into heat and light energy
(8) Chemical energy changes into heat, light and sound energy
Question 25. State the energy changes in the following case while in use:
1. loudspeaker
2. a steam engine
3. Microphone
4. washing machine
5. an electric bulb
6. burning coal
7. a solar cell
8. bio-gas burner
9. an electric cell in a circuit
10. a petrol engine of a running car
11. an electric toaster
12. a ceiling fan
13. an electromagnet.
Solution:
(1) Electrical energy into sound energy
(2) Heat energy into mechanical energy
(3) Sound energy into electrical energy
(4) Electrical energy to mechanical energy
(5) Electrical energy into light energy
(6) Chemical energy to heat energy
(7) Light energy into electrical energy
(8) Chemical energy into heat energy
(9) Chemical energy into electrical energy
(10) Chemical energy to mechanical energy
(11) Electrical energy into heat energy
(12) Electrical energy into mechanical energy
(13) Electrical energy into magnetic energy
Question 26. Name the process used for producing electricity using nuclear energy.
Solution:
The process used to transfer nuclear energy to electrical energy is called nuclear fission.
Question 27. Is it practically possible to convert a form of energy completely into the other useful form? Explain your answer.
Solution:
No. This is due to the fact that whenever energy is converted from one form to another, some of the energy also dissipates as heat that is lost to the environment.
Question 28. What is degraded energy?
Solution:
When energy is transformed from one form to another desired one, some of the energy is changed into another undesired form or is lost to the environment owing to friction or radiations, neither of which can be employed for any useful activity. This is referred to as energy dissipation, and this unwanted component of energy is referred to as degraded energy.
Question 29. What do you mean by degradation of energy? Explain it by taking one example of your daily life.
Solution:
Some energy is changed into undesired forms during the transfer of energy from one form to another desired one, or some energy is lost to the environment owing to friction or radiations, which cannot be employed for any beneficial purpose. This is referred to as energy dissipation or energy degeneration.
Example: When a light bulb is on, the electrical energy used is primarily transformed to heat energy, with some electrical energy also being turned to usable light energy.
Question 30. Complete the following sentence:
The conversion of part of energy into an undesirable form is called……………..
Solution:
Dissipation or degradation of energy refers to the transformation of a portion of energy into an unfavorable form.
Exercise -2(B)
Multiple Choice Type:–
Question 1. A body at a height possesses:
a) Kinetic energy
b) Potential energy
c) Solar energy
d) Heat energy
Solution: b) Potential energy
Question 2. In an electric cell which in use, the change in energy is from:
a) Electrical to mechanical
b) Electrical to chemical
c) Chemical to mechanical
d) Chemical to electrical
Solution: d) Chemical to electrical
Exercise -2(B)
Question 1. Two bodies of equal masses are placed at heights h and 2h. Find the ration of their gravitational potential energies.
Solution:
Height H1 = h
Height H2 = 2h
Mass of body 1 = m
Mass of body 2 = m
Gravitational potential energy of body 1 = mgH1 = mgh
Gravitational potential energy of Body 2 = mgH2 = mg (2h)

Question 2. Find the gravitational potential energy of 1 kg mass kept at a height of 5 m above the ground if g = 10 ms-2.
Solution:
Mass, m = 1kg
Height, h = 5m
Gravitational potential energy = mgh
= 1 × 10 × 5
= 50J
Question 3. A box of weight 150 kgf has gravitational potential energy stored in it equal to 14700 J. Find the height of the box above the ground. (Take g = 9.8 N kg-1)
Solution:
Gravitational potential energy = 14700 J
Force of gravity = mg = 150 × 9.8N/kg = 1470N
Gravitational potential energy = mgh
14700 = 1470 x h
h = 10m
Question 4. A body of mass 5 kg falls from a height of 10 m to 4 m. Calculate:
1. The loss in potential energy of the body
2. The total energy possessed by the body at any instant? (Take g = 10 ms-2).
Solution:
(1) Mass of the body = 5 kg
P.E. at height 10m = mgh = 5 × 10 × 10 = 500 J
P.E. at height 4 m = mgh = 5 × 10 × 4 = 200 J
Loss in P.E. = (500 – 200) J = 300 J
(2) The total energy possessed by the body at any instant remains constant for free-fall
It is equal to the sum of P.E. and K.E
At height 10 m, i.e. at top most point K.E = 0
Total energy = P.E. + K.E.
Total energy = 500 + 0 = 500 J
Question 5. Calculate the height through which a body of mass 0.5 kg is lifted if the energy spent in doing so is 1.0 J. Take g = 10 ms-2.
Solution:
Mass = 0.5 kg
Energy = 1 J
Gravitational potential energy = mgh
1 = 0.5 × 10 × h
1 = 5h
Height, h = 0.2 m
Question 6. A boy weighing 25 kgf climbs up from the first floor at height 3 m above the ground to the third floor at height 9m above the ground. What will be the increase in his gravitational potential energy? (Take g = 10 N kg-1)
Solution:
Force of gravity on boy = mg = 25 × 10 = 250N
Increase in gravitational potential energy = Mg (h2-h1)
= 250 × (9−3)
=250 × 6 = 1500 J
Question 7. A vessel containing 50 kg of water is placed at a height 15 m above the ground. Assuming the gravitational potential energy at ground to be zero, what will be the gravitational potential energy of water in the vessel? (g = 10 ms-2)
Solution:
Mass of water, m = 50kg
Height, h = 15m
Gravitational potential energy = mgh
= 50 × 10 × 15
= 7500 J
Question 8. A man of mass 50 kg climbs up a ladder of height 10 m. Calculate: (i) the work done by the man, (ii) the increase in his potential energy. (g = 9.8 ms-2)
Solution:
Mass of man = 50kg
Height of ladder, h2 = 10m
(i) Work done by man = mgh2
= 50 × 9.8 × 10 = 4900J
(ii) increase in his potential energy:
Height,h2 = 10m
Reference point is ground, h1 = 0m
Gravitational potential energy = Mg (h2-h1)
= 50 × 9.8 × (10-0)
= 50 × 9.8 × 10 = 4900J
Question 9. A block A, whose weight is 200 N, is pulled up a slope of length 5 m by means of a constant force F (= 150 N) as illustrated in Fig 2.13
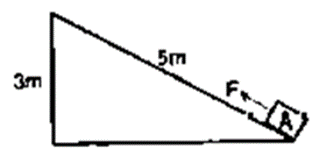
(a) What is the work done by the force F in moving the block A, 5 m along the slope?
(b) By how much has the potential energy of the block A increased?
(c) Account for the difference in work done by the force and the increase in potential energy of the block.
Solution:
F = 150N
(a) Work done by the force in moving the block 5m along the slope = Force x displacement in the direction of force = 150 × 5 =750 J
(b) The potential energy gained by the block
U = mgh where h = 3m
= 200 × 3 = 600 J
(c) The difference i.e., 150 J energy is used in doing work against friction between the block and the slope, which will appear as heat energy.
Question 10. Find the kinetic energy of a body of mass 1 kg moving with a uniform velocity of 10 ms-1.
Solution:
Mass, m = 1kg
Velocity, v = 10m/s
Kinetic energy = 1/2 ×𝑚𝑎𝑠𝑠 ×(𝑣𝑒𝑙𝑜𝑐𝑖𝑡𝑦)2
= 1/2 ×1 ×(10)2= 1/2 ×1 ×100
= 50J
Question 11. If the speed of a car is halved, how does its kinetic energy change?
Solution:
When the speed is cut in half while keeping the mass constant, the kinetic energy drops to one-fourth (since kinetic energy is proportional to the square of velocity).
Question 12. Calculate the decrease in the kinetic energy of a moving body if its velocity reduces to half of the initial velocity.
Solution:
Kinetic energy is directly proportional to the square of velocity.
Velocity is reduced to half its original value. So, we get
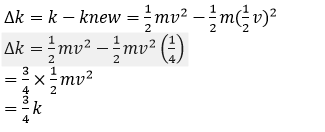
Question 13. Two bodies of equal masses are moving with uniform velocities v and 2v. Find the ratio of their kinetic energies.
Solution:
It is given that, the velocity of the first body v1 = v
And the velocity of second body, v2 = 2v
Since masses are same, kinetic energy is directly proportional to the square of the velocity (Kav2) Hence, ratio of their kinetic energies is:
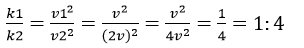
Question 14. A car is running at a speed of 15km h-1 while another similar car is moving at a speed of 30km h1. Find the ration of their kinetic energies.
Solution:
A car is running at a speed of 15 km h-1 while another similar car is moving at a speed of 30 km h-1. Find the ration of their kinetic energies
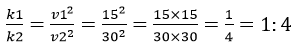
Question 15. A ball of mass 0.5 kg slows down from a speed of 5m/s-1 to that of 3m/s-1. Calculate the change in kinetic energy of the ball.
Solution:
Mass of ball= 0.5kg
Initial velocity=5m/s
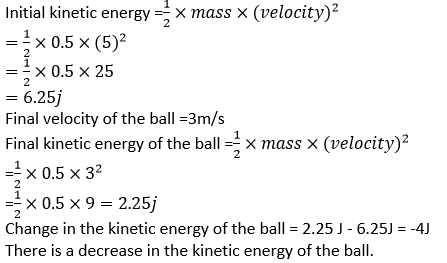
Question 16 .A cannon ball of mass 500 g is fired with a speed of 15 m s-. Find:
a) its kinetic energy
b) its momentum.
Solution:
(1) Mass of canon ball = 500g = 0.5 kg
Speed, v = 15m/s
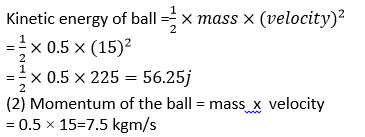
Question 17. A body of mass 10 kg is moving with a velocity 20 ms-1. If the mass of the body is doubled and its velocity is halved, find the ratio of the initial kinetic energy to the final kinetic energy.
Solution:
Let initial Mass, m1= 10kg and velocity, v1 = 20 m/s
Final mass, m2 = 2 × 10 = 20 kg and velocity, v2 = 20/2 = 10m/s
Initial kinetic energy, K1= 1/2 ×𝑚𝑎𝑠𝑠 ×(𝑣𝑒𝑙𝑜𝑐𝑖𝑡𝑦)2
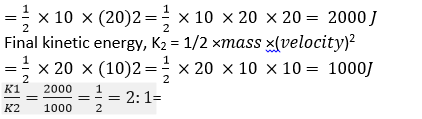
Question 18. A truck weighing 1000 kgf changes its speed from 36 km h-1 to 72 km h-1 in 2minutes. Calculate:
(i) the work done by the engine and
(ii) its power/ (g = 10 ms-2)
Solution:

Question 19. A body of mass 60 kg has the momentum 3000 kg ms-1. Calculate:
1. the kinetic energy
2. the speed of the body.
Solution:
Mass of body = 60 kg
Momentum, p = 3000kgm/s

(ii) Mass of body = 60 kg
Momentum, p=3000 kgm/s
Momentum = mass x velocity
3000 = 60 x velocity
Velocity = 50 m/s
Question 20. How much work is needed to be done on a ball of mass 50g to give it’s momentum of 500 g cms1?
Solution:
Momentum, p = 500 gcm/s = 0.005 kgm/s
Mass of ball = 50 g = 0.05kg
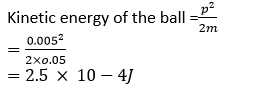
Question 21. How much energy is gained by a box of mass 20 kg when a man
1. Carrying the box waits for 5 minutes for a bus
2. Runs carrying the box with a speed of 3 m/s-1 to catch the bus
3. Raises the box by 0.5 m in order to place it inside the bus? (g=10 m/s-2)
Solution:
(1) Mass of box = 20 kg
Zero work is done as there is no displacement of the man.
(2) Mass of box=20 kg
Work done, Kinetic energy of man
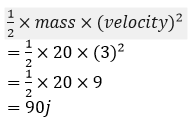
Question 22. A bullet of mass 50 g is moving with a velocity of 500 ms-1. It penetrated 10 cm into a still target and comes to rest. Calculate:
(a) the kinetic energy possessed by the bullet,
(b) the average retarding force offered by the target.
Solution:
Mass of bullet = 50g = 0.05kg
Velocity = 500m/s
Distance penetrated by the bullet = 10cm = 0.1m
(a) Kinetic energy of the bullet = 1/2 ×𝑚𝑎𝑠𝑠 ×(𝑣𝑒𝑙𝑜𝑐𝑖𝑡𝑦)2
= 1/2 ×0.05 ×(500)2
= 1/2 ×0.05 ×500 ×500=6250 J
(b) Work done by the bullet against the material of the target= resistive force x distance
6250 = resistive force × 0.1m
Resistive force = 62500 N
Question 23. A spring is kept compressed by a small trolley of mass 0.5 kg lying on a smooth horizontal surface as shown in the adjacent fig. when the trolley is released, it is found to move at a speed v = 2 ms-1. What potential energy did the spring possess when compressed?
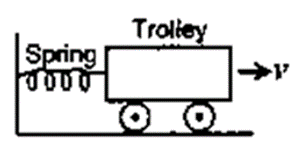
Solution:
Mass of trolley = 0.5 kg
Velocity = 2 m/s
When the compressed spring is released, its potential energy is converted into kinetic energy completely.
Potential energy of compressed spring = kinetic energy of moving trolley
Kinetic energy of trolley = 1/2 × mass × (velocity)2
= 1/2 ×0.5 ×(2)2
= 1/2 ×0.5 ×2×2=1J
Hence, potential energy of compressed spring = 1.0J
Exercise-2(C)
Question 1. State the law of conservation of energy.
Solution:
Energy cannot be generated or destroyed, according to the rule of conservation of energy. It only undergoes a single shape change.
Question 2. (1) What do you understand by the conservation of mechanical energy?
(2) State the condition under which the mechanical energy is conserved.
Solution:
(1) The rule of conservation of mechanical energy states that anytime there is a transfer of kinetic energy from one state to another, the total mechanical energy—that is, the sum of kinetic energy K and potential energy U—remains constant, or K + U = constant in the absence of frictional forces.
(2) For a given system, mechanical energy is only preserved when there are no frictional forces (i.e. between body and air). Therefore, the principle of mechanical energy conservation only applies in a vacuum, when air friction is completely eliminated.
Question 3. Name two examples in which the mechanical energy of a system remains constant.
Solution:
Motion of a free-falling body and a basic pendulum.
Question 4. A body is thrown vertically upwards. Its velocity keeps on decreasing. What happens to its kinetic energy as its velocity becomes zero?
Solution:
When a body is hurled vertically upward and its velocity is reduced to zero, its kinetic energy transforms into potential energy.
Question 5. A body falls freely under gravity from rest.
1. Name the kind of energy it will possess at the point from where it falls.
2. Name the kind of energy it will possess while falling.
3. Name the kind of energy it will possess on reaching the ground.
Solution:
(1) Potential energy.
(2) Potential energy and kinetic energy
(3) Kinetic energy
Question 6. Show that the sum of kinetic energy and potential energy (i.e., total mechanical energy) is always conserved in the case of a freely falling body under gravity (with air resistance neglected) from a height h by finding it when
(i) the body is at the top
(ii) the body has fallen a distance x,
(iii) the body has reached the ground.
Solution:
Let a body of mass m be falling freely under gravity from a height h above the ground (i.e., from position A). Let us now calculate the sum of kinetic energy K and potential energy U at various positions, say at A (at height h above the ground), at B (when it has fallen through a distance x) and at C (on the ground).
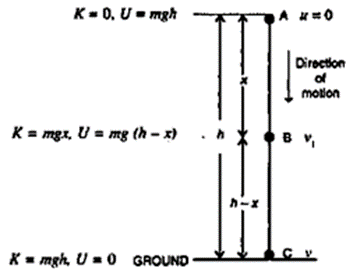
(i) At the position A (at height h above the ground):
Initial velocity of body= 0 (since body is at rest at A)
Hence, kinetic energy K =0
Potential energy U = mgh
Hence total energy = K + U = 0 + mgh = mgh .. —–(i)
(ii) At the position B (when it has fallen a distance x):
Let v1 be the velocity acquired by the body at B after falling through a distance x. Then u =
0, S = x, a = g
From equation v2 = u2 + 2As
v12= 0 +2gx =2gx
Hence, Kinetic energy K = 12mv21
Now at B, height of body above the ground = h – x
Hence, potential energy U = mg (h – x)
Hence total energy = K + U
= mgx + mg (h – x) = mgh ———– (ii)
(iii) At the position C (on the ground):
Let the velocity acquired by the body on reaching the ground be v. Then u = 0,
S = h, a = g
From equation: v2 = u2+2aS
v2 = 02 + 2gh
v2 = 2gh
Hence, kinetic energy K = 1/2 mv 2/1
=1/2𝑚 (2gh) = mgh
And potentiall energy U = 0 (a the ground when h = 0)
Hence total energy = K+U = mgh + 0 = mgh —— (iii)
Thus from equation (i), (ii) and (iii), we note that the total mechanical energy i.e., the sum of kinetic energy and potential energy always remain constant at each point of motion and is equal to initial potential energy at height h
Question 7. A pendulum is oscillating on either side of its resting position. Explain the energy changes that take place in the oscillating pendulum. How does the mechanical energy remain constant in it? Draw the necessary diagram.
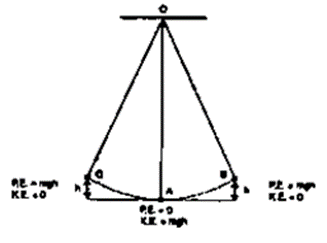
Solution:
The kinetic energy of the bob reduces as it moves from A to B, and its potential energy reaches its peak at B, where it is briefly at rest.
The process is repeated several times, with the potential energy switching back and forth from B to A. Thus, while swinging, the bob only has kinetic energy in the resting position A and potential energy only at the extreme position B or C. The bob possesses both kinetic and potential energy while it is at an intermediate position (between A and B or between A and C), and the sum of these two energies (i.e., the total mechanical energy) is constant throughout the swing.
Question 8. A pendulum with a bob of mass m is oscillating on either side from its resting position A between the extremes B and C at a vertical height h and A. what is the kinetic energy K and potential energy U when the pendulum is at position
(i) A,
(ii) B
(iii) C
Solution:
(a) The pendulum’s maximum kinetic energy is at point A, and its potential energy is zero at rest. K=mgh and U=0 as a result.
(b) At B, kinetic energy decreases and potential energy increases. Hence, K= 0 and U=mgh
(c) At C also, kinetic energy K= 0 and potential energy U=mgh.
Question 9. Name the type of energy possessed by the bob of a simple pendulum when it is
1. at the extreme position
2. at the mean position
3. it is at between the mean and extreme positions.
Solution:
(1) Extreme position: Potential energy
(2) Mean position: Kinetic energy
(3) Between mean and extreme: Both kinetic energy and potential energy
Question 10. (1) What is a solar cell?
(2) State whether a solar cell produces a.c. or d.c.
(3) Give one disadvantage of using a solar cell
Solution:
(1) A solar cell is a type of electrical device that uses the photovoltaic effect to transform light energy directly into electricity. Typically, semiconductors like silicon and gallium are used to create solar cells, along with certain impurities. When sunlight is shone upon a solar cell, a potential difference between its surfaces is created. As a result, a current flows in the circuit connected between the semiconductor’s opposing faces.
(2) DC is created by a solar cell.
(3) One disadvantage of solar cell is listed below:
The initial cost of a solar panel is sufficiently high.
Question 11. State two advantages of producing
1. electricity from solar energy.
2. electricity from solar energy.
Solution:
(1) Advantages of using solar panels:
1. They don’t pollute the environment in any way.
2. Solar panels practically never need to be maintained.
3. They endure for a very long time.
4. They don’t need to be maintained.
5. They work well in locations that are hard to reach and where power lines cannot be installed.
(2) Disadvantages of using solar panels:
1. A solar panel has a suitably high upfront cost.
2. Solar energy is inefficiently converted to electricity.
3. DC power generated by solar panels cannot be used for many domestic tasks directly.
Question 12. (1) What is wind energy?
(2) How is wind energy used to produce electricity.
(3) How much electric power is generated in India using the wing energy?
Solution:
(1) Wind energy is the kinetic energy of moving enormous quantities of air.
(2) A wind generator uses wind energy to generate electricity by using a wind mill to power the device.
(3) At the moment, wind energy is used to produce more than 1025MW of electricity in India.
Question 13. (1) State two advantages of using wind energy for generating electricity.
(2) State two disadvantages of using wind energy for generating electricity.
Solution:
(1) Advantages of using the wind energy:
1. It is pollution-free in every way.
2. It is an endless source, secondly.
(2) Disadvantages of using wind energy:
1. It costs money to set up a wind farm.
2. A substantial amount of land is required for the construction of a wind farm.
Question 14. (1) What is hydro energy?
(2) Explain the principle of generating electricity from hydro energy.
(3) How much hydroelectric power is generated in India?
Solution:
(1) Water or hydro energy is the kinetic energy that the flowing water has.
(2) The water flowing in high altitude rivers is gathered in a high dam according to the hydroelectric power plant’s basic operating principle (or reservoir). The water turbine, which is situated close to the bottom of the dam, is then given permission to receive the water from the dam. The armature of an electric generator or dynamo is linked to the shaft of the turbine.
(3) At the moment, just 23% of all electricity is produced via hydropower.
Question 15. (1) State two advantage of producing hydroelectricity.
(2) State two disadvantages of producing hydroelectricity.
Solution:
(1) Advantages of producing the hydroelectricity:
1. There is no environmental contamination as a result.
2. It is a renewable energy source, too.
(2) Disadvantages of producing hydroelectricity:
1. Due to the construction of dams over the rivers, plants and animals of that place get destroyed or killed.
2. The ecological balance in the downstream areas of rivers gets disturbed.
Question 16. (1) What is nuclear energy?
(2) Explain the principle of producing electricity using nuclear energy.
Solution:
(1) When a heavy nucleus is bombarded with slow neutrons, it splits into two nearly equal light nuclei with a release of tremendous amount of energy. In this process of nuclear fission, the total sum of masses of products is less than the total sum of masses of reactants. This lost mass gets converted into energy. The energy so released is called nuclear energy.
(2) Principle: The heat energy released due to the controlled chain reaction of nuclear fission of uranium-235 in a nuclear reactor is absorbed by the coolant which then passes through the coils of a heat exchanger containing water. The water in heat exchanger gets heated and converts into steam. The steam is used to rotate the turbine which in turn rotates the armature of a generator in a magnetic field and thus produces electricity.
Question 17. State the energy transformation on the following:
1. Electricity is obtained from solar energy.
2. Name two places in india where electricity is generated from nuclear power plants.
Solution:
(1) Light energy into electrical energy
(2) Tarapur in Maharashtra and Narora in Uttar Pradesh are the places where electricity is produced by use of nuclear energy.
Question 18. (1) State two advantages of using nuclear energy for producing electricity.
(2) State two disadvantages of using nuclear energy for producing electricity.
Solution:
(1) Advantages of using nuclear energy:
1. A very small amount of nuclear fuel can produce a tremendous amount of energy.
2. Once the nuclear fuel is loaded into nuclear power plant, it continues to release energy for several years.
(2) Disadvantages of using nuclear energy:
1. It is not a clean source of energy because very harmful nuclear radiations are produced in the process.
2. The waste causes environmental pollution.
Question 19. State the energy transformation on the following:
1. Electricity is obtained from solar energy.
2. Electricity is obtained from wind energy.
3. Electricity is obtained from hydro energy.
4. Electricity is obtained from nuclear energy
Solution:
(1) Light energy into electrical energy
(2) Mechanical energy into electrical energy.
(3) Mechanical energy into electrical energy.
(4) Nuclear energy (or heat energy) into electrical energy.
Question 20. State four ways for the judicious use of energy.
Solution:
Four ways for the judicious use of energy:
(a) The fossil fuels such as coal, petroleum, natural gas should be used only for the limited purposes when there is no other alternative source of energy available.
(b) The wastage of energy should be avoided.
(c) Efforts must be made to make use of energy for community or group purposes.
(d) The cutting of trees must be banned and more and more new trees must be roped to grow.
Exercise-2(C)
Question 1. A ball of mass m is thrown vertically up with an initial velocity so as to reach a height h. The correct statement is:
a) Potential energy of the ball at the ground is mgh.
b) Kinetic energy to the ball at the ground is zero.
c) Kinetic energy of the ball at the highest point is mgh.
d) Potential energy of the ball at the highest point is mgh.
Solution: a) Potential energy of the ball at the highest point is mgh.
Question 2. A pendulum is oscillating on either side of its rest position. The correct statement is:
a) It has only the kinetic energy at its each position.
b) It has the maximum kinetic energy at its extreme position.
c) It has the maximum potential energy at its mean position.
d) The sum of its kinetic and potential energy remains constant throughout the motion.
Solution: d) The sum of its kinetic and potential energy remains constant throughout the motion.
Exercise-2(C)
Question 1 A ball of mass 0.20 kg is thrown vertically upwards with an initial velocity of 20 ms-1. Calculate the maximum potential energy it gains as it goes up.
Solution:
Potential energy at the maximum height= initial kinetic energy
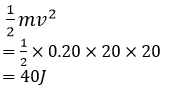
Question 2. A stone of mass 500g is thrown vertically upwards with a velocity of 15 m s-1. Calculate:
(a) the potential energy at the greatest height,
(b) the kinetic energy on reaching the ground,
(c) the total energy at its half-way point.
Solution:
(a) Potential energy at the greatest height = initial kinetic energy
or, mgh = 1/2 𝑚𝑣2
= 1/2 ×0.500 ×15 ×15=56.25J
(b) Kinetic energy on reaching the ground= potential energy at the greatest height = 56.25 J
(c) Total energy at its half-way point = 1/2 (K + U) = 56.25J
Question 3. A metal ball of mass 2 kg is allowed to fall freely from rest from a height of 5m above the ground.
(Take g = 10 ms-2)
1. Calculate the potential energy possessed by the ball when initially at rest.
2. What the kinetic energy of the ball just before it hits the ground
3. What happens to the mechanical energy after the ball hits the ground and comes to rest ?
Solution:
(1) Potential energy of the ball = mgh
= 2 x 10 x 5 =100J
(2) Kinetic energy of the ball just before hitting the ground =
Initial potential energy= mgh=2 × 10 × 5 = 100 J
(3) Mechanical energy converts into heat and sound energy.
Question 4. The diagram given below shows a ski jump. A skier weighing 60 kg stands at A at the top of ski jump. He moves from A to B and takes off for his jump at B.
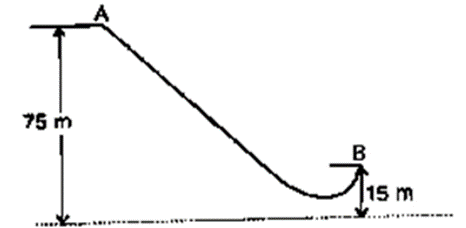
(a) Calculate the change in the gravitational potential energy of the skier between A and B.
(b) If 75% of the energy in part (a) becomes kinetic energy at B. Calculate the speed at which the skier arrives at B.
(Take g=10 ms-2)
Solution:
It is given that, Mass of skier= 60kg
(a) Loss in potential energy = mg(h1 –h2)
= 60 × 10 × (75 −15)
= 60 × 10 × 60 = 3.6 × 104J
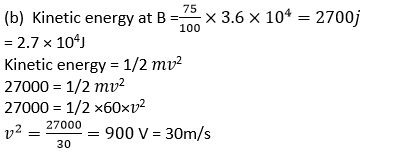
Question 5. A hydroelectric power station takes its water from a lake whose water level if 50 m above the turbine. Assuming an overall efficiency of 40%, calculate the mass of water which must flow through the turbine each second to produce power output of 1 MV.
Solution:
Potential energy = mgh
Efficiency = 40%
Useful work done = 40% of potential energy

Question 6 The bob of a simple pendulum is imparted a velocity 5 m s-1 when it is at its mean position. To what maximum vertical height will it rise on reaching to its extreme position if 60% of its energy is lost in overcome friction of air?
Solution:
Potential energy at the extreme position = 40% of kinetic energy at the resting position.